
D. H. GIESKIENG, WOFK, 9653 Rensselaer Drive, Arvada, Colorado 80004 and H. ASPDEN, FIEE, Department of Electrical Engineering, University of Southampton, Southampton SO9 SNH, England.
Antennas designed to radiate electric and magnetic fields in quadrature time-phase are found to have anomalous radiation properties relative to the in-phase propagation properties of the conventional dipole. It is shown that there is a marked advantage in wave survival efficiency over the dipole, increasingly evident beyond a mile range. This is attributed to the excitation of a natural wave propagation mode by the new antenna, rather than the dipole's forced wave propagation mode and the deceleration of the latter over the short range into a natural wave with some energy dissipation.
In summary, the Gieskieng antenna differs from conventional antennas in that it is expressly designed so that the electric field energy and the magnetic field energy are not forced into the field medium in time-phase with each other, as applies for a conventional half-wave dipole. Instead, the antenna is so structured that the electric and magnetic fields are set up in quadrature phase. For the same excitation this appears to produce a signal of half strength initially, but it has, it seems, the property of being less subject to dissipation and attenuates more in accord with theoretical prediction, whereas conventionally produced signals attentuate more rapidly.
It will be argued that this phenomenon is attributable to the field medium having a natural propagation mode which is directly excited by the Gieskieng antenna, whereas the excitation by the dipole antenna develops a forced propagation mode with which we are familiar, the forced propagation degenerating into natural mode propagation with energy dissipation over an initial range of transmission.
Gieskieng [1] disclosed the features of his new antenna in a short note published in The Mines Magazine in 1981 and interpreted its unusual properties as due to a separation of the truly electromagnetic radiation from that associated with the electric field. Such argument was tentative, as may be the theoretical argument now offered below. The essential point is the appreciation that there is an anomaly involved in the antenna radiation properties and that an answer must be found in the interests of communication technology. The experimental findings reported here are, therefore, the more important element of this paper. However, since measurements of the kind reported are notoriously difficult and it is, therefore, easy to doubt their validity, some elaboration of the theoretical background relating to wave propagation, seems in order. In this way it is hoped that the reader may see the results in context and favour further research into what does appear to be a quite fundamental avenue for development.
Maxwell's equations are silent on the subject of such threshold frequency conditions, but it is clear that something endows the vacuum medium with a resonant property at the Compton electron frequency 1.24 1020 Hz.
Historically, Maxwell's equations had a physical basis. They relied upon the so-called displacement current and belief in the ether as a tangible medium filling space. This was before Compton's discovery of the wave interaction with the electron. The measured isotropy of light speed led to the ether going out of fashion. Displacement currents were retained only within the mathematical framework of Maxwell's equations, which, however, gave no physical picture from which to assess threshold conditions. The ether has been revived by quantum techniques. The energy fluctuations known to exist in the vacuum and the quantum interactions which form the basis of quantum-electrodynamics and electron-positron pair creation and annihilation have made the ether respectable again, but in a new adaptable form. Graham and Lahoz [2] have gone further in demonstrating that the vacuum can be the seat of electrically-induced inertial reactions and have affirmed from their experiments that Maxwell's displacement currents are real properties. Maxwell's equations warrant rescrutiny in these circumstances.
There are of historic record proposals for generalizing Maxwell's equations in order to accommodate for the motion of the ether with an observer. Hertz [3] postulated the need for total time derivative formulations, a theme followed recently by Tombe [4], who suggests that the partial derivative form of Maxwell's equations prevents them from being Galilean invariant and proposes corrections modifying the equations. Tombe refers to independent proposals along the same lines by Phipps [5] and Kosowski [6]. Also of record in connection with efforts to adjust the equations to conform with speed anisotropy observations are the modifications proposed by Trimmer and Baierlein [7] which retain the partial derivative form but introduce small anisotropy constants connected with a preferred direction in space.
Such modifications of the fundamental equations which have proved quite well founded must be seen as speculative, pending experimental evidence stronger than that now available. Here, we do have some experimental evidence, but it strikes at something more basic than the equations themselves, that is the energy transfer issue not really embraced by the field equations as formulated.
The points at issue, theoretically, are the energy transfer process, the threshold frequency condition and, a rather unusual question concerning the nature of a magnetic field in a wave propagating through the free vacuum. If the field arises from electric displacement currents, there is the question of what is actually displaced and relative to what is it displaced? We avoid these questions, and perhaps miss technological opportunities, if we look only at Maxwell's equations.
Considering the threshold frequency condition and the prospect from the above-mentioned experiments by Graham and Lahoz that a mass property must be associated with the vacuum medium, Aspden [8] has shown that the displacement is a relative displacement. There are two constituents in the field medium which move in opposition when an electromagnetic wave is in transit in a natural propagation mode. It is only by this means that the medium can have an inertial property which is hidden from us under normal circumstances and does not cause frequency dispersion in long range wave propagation. The same principles have recently been shown to give basis for calculation of the small but progressive reduction of frequency associated with the red shift of modern cosmology. The Hubble constant was found to be in full accord with the observed value, without recourse to the expanding universe philosophy [9]. This endorses the proposition that we should look to the foundations of Maxwell's equations for new properties affecting electromagnetic wave transfer.
The energy transfer question is crucial and here we find that there is, of record, a quite challenging account in the work of Professor G. H. Livens, Fellow of Jesus College, Cambridge, England. In his book 'The Theory of Electricity', 2nd. Ed. published by Cambridge University Press in 1926, under the heading 'On the flux of energy in radiation fields', he wrote:
'The usual procedure is to base the whole of the discussion on Poynting's form of the theory, which appears to provide the simplest view of the phenomena, and to ignore the possibility of alternatives. We must not however forget that our viewpoint may be coloured by a long use of the particular form of the theory as the sole possibility, so that its apparent suitability may be misleading. It is therefore essential that we bear in mind that Poynting's theory is not the only one which is consistent with the rest of the electromagnetic scheme.'
Liven's then demonstrated that all the empirical evidence was also consistent with the field oscillations being established by energy stored in the kinetic energy of the field in the proximity of the antenna and sustained in propagation without energy transfer at the wave velocity. When waves are intercepted the same energy is absorbed as one calculates from conventional theory based on Poynting's assumptions. In a sense, therefore, we have here, in 1926, proposals which could well be compatible with the waves acting as catalysts in promoting quantum energy transfer from a symmetrical energy-primed background, a theme later developed in quantum physics. Liven's work is extensively discussed in a 1972 book by Aspden [10].
When a standard half-wave dipole is excited the electric field oscillation is set up as shown in Fig. 1 and propagates at the speed c in the direction shown by the arrow. The field medium has a response time of the order of 10-20 s and will follow these field oscillations with negligible dispersion. The related dipole current sets up a magnetic field oscillation in time phase with the electric field but in space quadrature. Energy is, therefore, forced into the waves, shared equally between the electric and magnetic fields, and must travel at speed c. Maxwell's equations assure that the electric and magnetic waves are mutually sustained. In energy terms, we expect that energy from the electric field transfers forward to the magnetic field and vice versa.

The above is conventional. Now we add our constraints. First, if the field medium has a natural threshold frequency and so a related inertial property, then such a wave must eventually suffer frequency dispersion. Yet, in the true vacuum that we associate with outer space, Warner and Nather [11] have found that the group velocity of light is constant to better than 5 parts in 1017. We must, therefore, at least have a suspicion that the accepted form of wave oscillation shown in Fig. 1 is not of the type which really does penetrate the vast distances of interstellar space. In theory, if we admit that a real vacuum medium is involved and are looking for technological consequences, we are forced to the view that such a wave must be degenerate.
Now we ask if a wave can be produced without forcing the magnetic field to be in phase with the electric field, a condition that will be a deliberate design constraint of the antenna to be described. Note here that a magnetic field requires charge to move relative to the electromagnetic reference frame. According to modern relativistic principles, the latter is the observer's reference frame, but for waves in outer space this requirement stretches the imagination. On conventional old fashioned theory, this frame is set by the ether itself. So, either way, one wonders how, in true vacuum, there can be such a thing as a magnetic field. The thermodynamic characteristics of a magnetic field suggest reaction effects (see Aspden [12]) within a primary medium. Therefore, in the absence of matter, the basic symmetry of the primary vacuum medium to which it owes its transparency must be broken to create field substance sustaining the magnetic energy condition. This is a forced and unnatural state ultimately incurring dissipation of energy, but one which can no doubt exist, in view of the conventional type of wave depicted in Fig. 1.
In any event, there is good reason for accepting that the magnetic property is a special state of the field medium in which the kinetic energy of charge in a motion reacting to the inducing action appears to constitute what is, in a thermodynamic sense, the magnetic field energy.
The Gieskieng antenna to be described produces the electric field wave with a 90o time phase advance compared with the magnetic field wave, because in relation to the direction of wave propagation the electric dipoles are set one quarter wavelength ahead of the section producing the magnetic effect. This wave system is shown in Fig. 2.

The magnetic field H, shown in Fig. 2, may, therefore, be a notional field signifying dynamic energy in the field medium. On such an interpretation, Maxwell's field equations change from the form:
The proposition is that it is possible for the field medium to be set in a natural propagation mode which conforms with equations (3) and (4), so far as analogy with Maxwell's equations are concerned. These new equations are symmetrical, a favourable contrast with the conventional equations of (1) and (2), if they are to represent conditions in the vacuum medium not governed by the forced constraints of action involving matter.
The physical process involved in generating the Fig. 2 wave is that as energy is supplied into the electric field we do not drive energy into the magnetic field at the same rate but rather leave it to the electric field displacement to do all the work. The inductive effect of changing magnetic field does not then develop the electromotive forces which oppose and so contain the displacement. The result is that, for the same electric field, the Fig. 2 wave involves a much larger physical displacement of the field medium than does the Fig. 1 wave. The kinetic energy represented by the magnetic field is then primarily energy of the motion of the electric displacement, a much slower process than the one involved in the Fig. 1 wave. Indeed, there is a counter-displacement which offsets the electric field condition and adapts to the frequency of the signal in transit so as always to be in resonance at a level set by the energy density of the wave. This resonance is not excited in the forced wave mode of Fig. 1.
This process is justified elsewhere [8, 9], but the outline above will serve to show that we may expect unusual properties to be displayed by an antenna excited in the special mode just discussed. In particular, the Fig. 2 wave propagation mode should be subject to no dispersion and so should have better long range characteristics.
Two other points should be mentioned at this stage. The first is that a wave generated in the Fig. 1 mode will tend to degenerate into the Fig. 2 mode as the electric field oscillations progressively need to transfer energy into the kinetic form because the magnetic energy is slowly dissipated and cannot sustain the back EMFs which absorb energy from the electric field. Secondly, it is of interest to note that at zero frequency the counter-displacement state mentioned above is wholly balanced against any forward displacement. This is the state for which the vacuum medium has adapted to the local frame of the notional Earth-bound observer and moves with him through the cosmic space. However, in radio propagation we are concerned with the lateral displacements and these always have a partial, though nearly equal, electrical counterbalance displacement. Only at ultra high frequencies does this counter displacement become small, being zero at the threshold at which electrons and positrons are created.
The results are deemed to confirm the theory presented above, but it is emphasized that the data provided by these experiments was of record before the above theory was related to the specific findings. It is, therefore, quite rewarding to find that the extensive work involved in collecting the data has given results which stand up well against a theoretical background.
Further experiment will, no doubt, give additional confirmation. As noted in the earlier publication (Gieskieng [1]), experiments using the special antenna as a receiver have shown it to have significant advantages, as if it is particularly adapted to the natural wave mode which all radio signals degenerate into, according to the above proposition. Experiments have already been performed to set up interference tests between two transmitting antenna, firstly dipole versus the special antenna and secondly special versus special antenna. With the dipole interference the data show a progressive and predictable change of wavelength as between signal peaks close to the dipole (about ten wavelengths distant). However, with the interference between the two special antenna, the peak to peak distance does not change and so is not affected at this range by the direct wave components we associate with the dipole. This, itself, shows that we are dealing with a special wave mode propagation. These interference experiments are the subject of continuing research and will be reported on separately in due course.
The new antenna has been termed the 'Maxwell Antenna' by Gieskieng in his prior work, partly because of the emphasis placed upon the pure electromagnetic wave propagation properties and magnetic, as opposed to electric, content of Gieskieng's tentative interpretation as to its operation. In view of the new emphasis in this paper upon its operation as an electro-kinetic wave propagator, it seems preferable to label it with the name of its creator. Hence the use of the term 'Gieskieng Antenna' in the onward description.

It is a transmission line stub antenna having two legs of length B and a shorting bar of length A connecting adjacent ends of the two legs. In the form used in the tests described it was fabricated from tubing of diameter C. At the free ends there are tuning sleeves and there is a balun feed adjacent the shorting bar. The design requires the overall length 2B+A to be about a half wavelength of the signal to which the antenna is tuned. Suggested dimensions to cover the following amateur bands are as tabulated:
| Band | A | B | C |
|---|---|---|---|
| 20 mtr | 48 | 180 | 4 |
| 15 mtr | 38 | 133 | 3 |
| 10 mtr | 30 | 84 | 3 |
| 6 mtr | 18 | 45 | 2 |
| 2 mtr | 7 | 17.25 | 2 |
The lengths of A, B and C are given in inches.
The 145 MHz comparisons were made at a height of several wavelengths in addition to being on the edge of large cliffs, where both antennas could radiate with a great deal of certainty that the forward radiations would have negligible involvement with immediate ground and that when the downward portion of the wave did finally reflect it would be prevented from reaching the monitoring antenna by placing the latter some 300 feet back from the edge of the opposite cliff.
It was found impractical to make similar cliff-side tests at 14 MHz and the new antenna was therefore tested against existing beams, using a mountain-top Monitor station.
The following is a synopsis of some of the tests which were performed over a period of four years with the help of many colleagues (see later acknowledgement).
In this case, to provide the standard of reference, six existing commercially made triband Yagi beams and one monoband Yagi beam in the Golden-Arvada-Denver area were also tested to Squaw Mountain, with several checks in their favoured direction to assure obtaining their peak values. To provide a common basis of comparison their signal readings were adjusted to a range of 24 miles and further adjusted for lobe angle so as to provide a common reference. The latter correction was necessary because the monitor station on the top of Squaw Mountain only subtended vertical angles ranging from 2.0o to 3.33o from the test sites, whereas the lobes of the beams used peak at vertical angles ranging from 12o to 37o.
Both Yagi and Gieskieng antenna results are summarized in Fig. 4, where the average of the polarization components are shown. The curves relate the different Yagi beams according to their antenna height above ground and the Gieskieng antenna, in both vertical and horizontal positions, also for different heights. This result is best interpreted in the light of the further tests reported below.
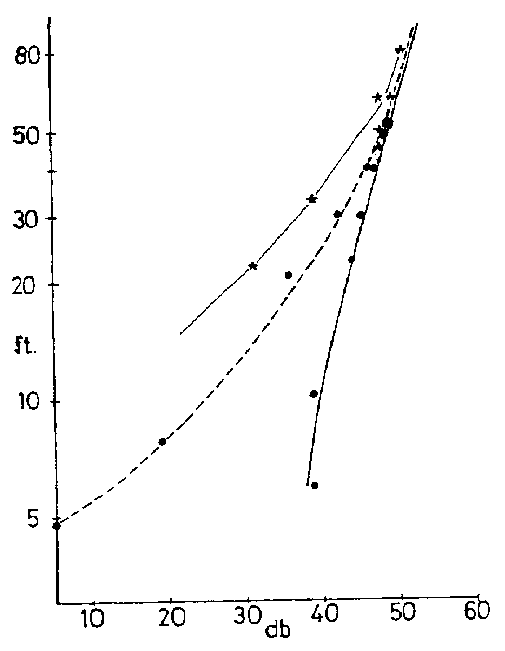
Fig. 4. Power averages of vertical and horizontal monitor antennas Yagi beam stations (*) and the Gieskieng antenna in horizontal mode (broken line) and vertical mode (full line), all related to antenna height above ground.
First, the Gieskieng antenna was rotated to Squaw Mountain to test its directional properties. It was found to be omnidirectional within about 1 db, confirming a result found for the test group 1 data, and then left in a fixed position. The owners of the two stations then undertook a closely-monitored comparison between the Gieskieng antenna and the two element quad (both being horizontally polarized). An analysis of the log of 17 stateside and worldwide mutual contacts, including reports on a Yagi that joined in on 9 of the contacts, gave 26 long range comparisons of antenna performance.
The contacts reported results for the quad and Yagi beams that were on average 4.5 db over the Gieskieng antenna performance. However, half of the 26 contacts reported precisely equal performance for the Gieskieng antenna, indicating that, in these instances, the latter has, approximately, a 3 db advantage over a dipole in long range wave survival efficiency.
The reason for this is understood if we compare performance as adjusted for isotropic radiation. The dipole lobe is 2.2 db over this reference level and the calculation of lobe off-centre power levels for the highest (80 ft) Yagi beam in the Fig. 4 data indicated that the beam lobe was only 0.75 db over the dipole. The Yagi beam should, on this basis, have about a 3 db advantage over the omnidirectional Gieskieng antenna. Yet, as Fig. 4 shows, the Yagi beams gave about the same results as the Gieskieng antenna, showing that the omnidirectional power of the latter could match the lobe power of the Yagi beam and had a 3 db advantage in wave survival efficiency. The same result was confirmed for half the 26 contacts of test group 2.
Subsequently, experiments at 2 meters made it possible to explore higher vertical angles by using a Gieskieng antenna to monitor the OSCAR satellite beacon. Passages above 80o still failed to indicate an overhead null. Thus the pattern of the antenna is regarded as being very nearly spherical (see later discussion).
Dipoles constructed of 12 gauge wire, 1.25 inch and 2.5 inch tubing were tested in the transmitting mode with a complete horizontal plane revolution for each of the receiving antenna side inclinations. The test group 1 work had shown that it was imperative to procure the average of at least the vertical and horizontal energy components to obtain consistent figures of merit and obtaining three additional intermediate components was a further refinement.
Immediately following the dipole tests, Gieskieng antennas constructed of 5/8 inch, l.25 inch and 2 inch diameter conductors were similarly tested. It should be noted that the 2 inch and 1.25 inch Gieskieng antennas were fed with untuned ferrite baluns, which provide symmetry but occasion some loss at 145 MHz. This loss was determined to be about 1 db and this was allowed for in the data presented for these two antennas. Subsequent tests using a 'bazooka' feed to eliminate cable contribution and to avoid the toroid loss verified this adjustment to within 0.2 db.
The test site was the Golden, Colorado area which has two large equally high basalt-capped plateaus, North and South Table Mountains, which are nearly one mile apart, have precipitous cliffs and are joined by a flat valley some 600 ft. below. This terrain provided an ideal opportunity to let the 2 meter transmitting antenna located near the edge of one of these cliffs radiate freely, in the knowledge that the earth reflections would be effectively blocked from reaching the receiving antenna, set back some 300 ft. from the edge of the other Table Mountain. The transmitting antenna was on a 16 ft. pole and the receiving antenna on a 20 ft. pole.
| Antenna | Horiz | 22.5o | 45o | 67.5o | 90o | Mean | Feed |
|---|---|---|---|---|---|---|---|
| G (2 in) | 18.99 | 17.73 | 14.90 | 10.04 | 2.44 | 14.53 | (1) |
| G (1.25 in) | 18.08 | 17.92 | 16.12 | 10.43 | 3.14 | 14.62 | (1) |
| G (5/8 in) | 18.37 | 17.99 | 15.35 | 10.38 | 2.97 | 14.58 | (2) |
| Mean | 18.48 | 17.88 | 15.46 | 10.28 | 2.85 | 14.58 | |
| D (2.5 in) | 16.90 | 16.60 | 13.75 | 8.76 | 3.09 | 13.18 | (3) |
| D (1.25 in) | 16.76 | 13.27 | 0.91 | 12.46 | (3) | ||
| D (12 ga) | 16.91 | 15.26 | 12.66 | 6.88 | 2.38 | 12.30 | (3) |
| D (12 ga) | 16.62 | 15.89 | 13.37 | 8.29 | 0.29 | 12.59 | (4) |
| Mean | 16.81 | 15.92 | 13.29 | 8.05 | 2.08 | 12.71 | |
| G - D | 1.67 | 1.96 | 2.17 | 2.23 | 0.77 | 1.87 |
The table shows signal strength in db for the different Gieskieng (G) and Dipole (D) antennas. It shows how a reading for a set position of the transmitting antennas is obtained. The average for the antennas in this particular position is 14.58 db for the Gs and 12.71 db for the Ds, given a mean advantage of the Gieskieng antenna over the dipole antenna of 1.87 db at the one mile range.

Fig. 5. Comparison of signal strengths from Gieskieng antennas and dipole antennas for receiving antenna side tilts of 0o (horizontal) (dotted line points), 22.5o (crosses on broken lines) and 45o (circles on lines).
The feeds used in Table II are (1) untuned balun, (2) untuned gamma, (3) tuned gamma and (4) double tuned balun.
The effect of rotating the transmitting antenna is shown in Fig. 5. This confirms the omnidirectional properties of the Gieskieng antenna in the horizontal plane and shows its power advantage over the equally energized dipole antenna.
The data obtained from these various tests are summarized in Fig. 6. The curve shows the measured power of the dipole antenna signal relative to that of the Gieskieng antenna, the five data points marked by dots being the comparisons at 145 MHz and corresponding to test groups 7, 6, 5, 4 and 8, respectively. The + data point was for the 14 MHz test of test group I and the * data point is the one inferred by the method of test group 2.

Fig. 6. Comparison of signal strength of dipole transmission in relation to that of equally powered Gieskieng antenna taken as 0 db ordinate reference.
The omnidirectional properties of the Gieskieng antenna are of special significance. If, as implied by the theoretical introduction, this antenna produces a displacement oscillation in the field medium which is a true natural oscillation in a direction parallel to the free ends of the antenna legs then it is logical that the wave radiating from it should be symmetrical about this axis. Radiation should therefore be isotropic in a transverse plane. However, radiation perpendicular to this plane will-also occur owing to the current in the two legs. This current is in anti-phase in these legs and, owing to their separation by the distance A in Fig. 3, a wave representative of the spacing will be propagated as a forced wave in the antenna plane and in a direction parallel with the shorting bar A. Since the legs are longer than the shorting bar, the fact that A is much smaller than a half wavelength will tend to be balanced and a somewhat spherically-symmetrical wave propagation can be expected, at least over a short range.
It is submitted that the data in Fig. 6 bears out the proposition that, whereas the Gieskieng antenna, radiates what is essentially a natural non-dispersive electromagnetic wave oscillation, the dipole antenna radiation is dissipated over a range of a few miles as half of its power is lost in adjusting to the natural propagation mode. The dipole antennas used in the tests would then respond at 50% efficiency at the longer range for the dipole and at near range and longer range for the Gieskieng antenna, thereby explaining the curve in Fig. 6.
Further research is needed to verify that if a Gieskieng antenna had been used as the receiving monitor in the tests, the transmitting Gieskieng antenna would have a four to one advantage over the dipole.
It is recognized that tests comparing theoretical propagation properties of antennas with their actual propagation properties are very difficult and hardly practical. See for example the work of Dolle and Cory [13] who compared radiation from a dipole antenna and a loop antenna at various frequencies and various close ranges. Above 100 MHz they found that the field attenuation measurements were erratic because they were influenced by nearby objects. However, though they did find that the dipole field attenuated more rapidly than theory predicted, other than obtaining some useful empirical data nothing of fundamental significance can be attached to the discrepancy.
It is submitted, therefore, that the tests reported here are rather special in the way in which the ground reflection problems are overcome and in the way an entirely new type of antenna forms part of the comparison.
If one considers whether tests could be performed on wave propagation under more controlled conditions, the question is then raised as to whether a pure sinusoidal signal propagated along a coaxial cable would degenerate in transit from a forced wave mode to a natural wave mode. On the theory presented it seems probable that the phase of the electric wave field will alter by one eighth of a wavelength over a range of adjustment (of the order of a few miles) because the in-phase electric and magnetic oscillations (Fig. 1) adjust to a quadrature phase relationship (Fig. 2). The question then arises, firstly, whether this, in fact, occurs and, secondly, if it does, what determines the rate of degradation of the signal? Such an experiment seems viable but, happily, it seems that this first possibility has already been tested and found affirmative. Torr and Kolen [4] have reported some very perplexing results in an experiment sending a 5 MHz signal along a 500 meter coaxial line. They sought to measure the one way speed of propagation, or rather its variation, by sending the signal between two atomic clocks and keeping track of its phase change. Assuming that the phase change measured was solely due to variations in propagation speed they inferred that the speed of light could vary in a one way measurement by as much as 1%. Phase differences of 8 nanoseconds or 0.04 wavelengths were found and they had a spurious dependence upon the time of day, an implication that there might be some sensitivity to atmospheric conditions. However, a change of wavelength of 0.04 in 500 meters would correspond with phase shift accompanying the attenuation predicted by this paper and apply over a range commensurate with the measurements reported in Fig. 6.
It follows, therefore, that the antenna data reported here may have independent support and relevance to basic research on coaxial cable transmissions. Torr and Kolen admitted being perplexed by their findings and drew attention to their dilemma by saying:
Since there is no theory available which can account for these variations, we believe that it is essential to repeat the experiment with different clocks ....
A theory has been provided in this work, but it remains to be seen whether it will stand the test of time. Meanwhile, however, there is purpose in exploiting the new antenna proposed and investigating the reasons for the degeneration of the forced Maxwell wave by studying wave interference phenomena.
Over 25 others came, in some cases repeatedly and from over considerable distances to help in manning the field stations, in loaning equipment, use of their towers and stations for the quad comparison tests as well as providing photographs of several field tests. This assistance included also the provision and manning of a balloon (Don Ida) for the flyover tests, provision of a computer and considerable time in integrating the Table Mountain tests and expert advice in antenna performance evaluations.
Readers may appreciate that what has been discussed in this paper and its introduction as provided by Lecture No 10 has very far reaching implications. The issue concerns the latent energy condition of the aether and it offers scope for reinterpretation of cosmological redshift data to avoid the absurdity of the belief that the universe was born at a single point in space and has been expanding ever since. It gives scope for a new interpretation of the source of heat which powers the sun. Indeed, at the end of the day, a day sometime in the 21st century, we will see that 'cold fusion' in its broadest sense will feature in the recognized forces of creation, the materialization of matter from the energy residing in the aether. These Web pages have much to report on this author's contribution to this subject.
*******