
The Universe began as a tiny particle, Stephen Hawking, the scientist and bestselling author has concluded. The Lucasian Professor of Mathematics at Cambridge University has turned his attention to what may have happened in the fraction of a second before Big Bang. Professor Hawking and Neil Turok, also of Cambridge, believe that not only was there a microscopic particle but that it was expanding in a process known as inflation just before Big Bang ...... Their theory, based on one put forward by Professor Hawking in 1983 and Einstein's theory of gravity, also concludes that the universe will expand for ever. .... The theory pointed to the Universe being cone-shaped, having started out as a dot in space and time and expanding like an ice-cream cornet over the 12 billion years. Professor Hawking will outline the theory at a meeting next month at the California Institute of Technology.
Now, it is extremely easy, and involves quite elementary physics, to prove that the universe, governed as it is by the standard physics of electric fields, is subject to the space constraints imposed by planar boundaries. Were this not so, the energy density stored by an electric field would not conform with the established empirical formulations of fundamental physics. This precludes the evolution of a universe, one which necessarily embraces the field medium associated with its electric fields, as expanding from a point, even in a symmetric spherical expansion. Furthermore, one must challenge the very belief that the universe expands, given that it is based on the assumption that light waves once emitted by a radiating atom never ever suffer a loss of frequency in their billions of years of passage through space. I have discussed this latter point in the previous lecture, Lecture No. 11, but I will seek in this Lecture No. 12 to clarify what I have just said about the planar space boundary.
S. W. Hawking
University of Cambridge
Department of Applied Mathematics and Theoretical Physics
Silver Street
Cambridge CB3 9EW
England
Dear Mr. Hawking:
Thank you for the copy of your paper, THE LIMITS OF SPACE AND TIME. One of the central themes appears to be gravity, and I thought that you might be interested in a paper I wrote some time ago, DOES AETHER CAUSE GRAVITY? It would seem to be the only medium universal enough to accommodate both radio and gravitation.
Harold Aspden of Southampton was kind enough to review my paper that you have, and suggested that I might write a brief paper, concentrating upon one of its graphs. This has just been done with rather startling perceptions of the aether and electric fields emerging. It is two pages, and titled: A CHARACTERIZATION OF THE AETHER.
In my previous efforts on this I have not been able to get the radio magazines interested in the antenna and its results. Since you have had some success with the more scientific publication, Nature, I am wondering if you would care to perhaps join in critiquing it for submittal to them. Would you have any other suggestions?
Yours sincerely,
(signed)
D. H. Gieskieng
9653 Renselaer Dr.
Arvada, Colorado 80004
U.S.A.
Now, let me say at once that I am not in the least surprised that this letter made no impact of Professor Hawking. Hawking is wedded to Einstein's theory and the Big Bang. He is hardly likely to be interested in anything which implies interest in the 'aether' and he is a theoretical physicist, with a cosmological speciality, so experiments involving radio antenna are unlikely to evoke his interest. Nor, indeed, are such letters from non-academic sources likely to be given more than a cursory glance. That said, however, the point is made that the experimental evidence which I see as a killer, so far as Big Bang theory is concerned, is there in those Gieskieng experiments. The aether, the non-expanding aether, with its energy attributes and its planar boundaries governing how electromagnetic waves shed energy to rely on aether energy, is playing its part in those experiments by Dave Gieskieng.
We know that most of that energy is stored in that something that we admit occupies the so-called vacuum. Teachers avoid reference to it as 'the aether'. They prefer to assign a dielectric constant to the vacuum and then tell us that there is really nothing there inside that vacuum. If we define the vacuum as 'space devoid of matter' then that does not preclude the presence of that 'something', which I say is the 'aether'. The teacher avoids the word and, if pressed, hides behind a screen of mathematics representing Maxwell's equations, but a discerning student knows that there is an aether, whatever the textbooks may say. However, I am sure that some students must be so disillusioned by the lack of physical reality and reliance of mere mathematical formulations, all arising from the aether being 'outlawed' that some, at least, must turn away from physics and, as a result, wander into other fields of learning.
There is an aether! To store energy in that aether, in accordance with the facts observed by experiment, it must have planar boundaries. A parallel plate capacitor has such boundaries, but take away the metal plates and, somehow, somewhere in the space we inhabit, there exists those boundaries as a property of space itself. Otherwise, the energy density stored in the vacuum medium by an electric field would not conform with the formulae that we know are valid.
There is really no sense in imagining that the material universe can begin as a point and then spread to infinity, if one admits there is an aether present that already extends to infinity. What is the sense in trying to create a universe from 'nothing' - a mere point in space - when common sense tells us that if there is an aether present thoughout space, that aether is the likely source of the energy which feeds the creation of the universe? The universe should be associated with matter which is created throughout space and merely nucleates to form stars everywhere in space. So, to talk about expansion of the universe from a point one needs to explain how energy drawn from the aether ever converged on that point or bring the aether itself into the expansion scheme.
I wonder if Professor Hawking has even thought of this, because it is rather basic and it bears heavily upon his conclusions concerning the expanding form of the universe. The chances are that for 'aether' he substitutes Einstein's mathematical equations and it is then rather difficult to separate an argument about the 'universe' and whatever it is that those equations represent.
I will try not to comment further in this style and will, instead, concentrate on merely presenting some additional background information and then the formal elementary proof of the planar space boundary proposition.
Writing about the meaning of his General Theory, I see on page 107 of that book by Einstein a set of conclusions which read:
Thus we may present the following arguments against the conception of a space-infinite, and for the conception of a space-bounded, or closed, universe:-
1. From the standpoint of the theory of relativity, to postulate a closed universe is very much simpler than to postulate the corresponding boundary condition at infinity of the quasi-Euclidean structure of the universe.
2. The idea that Mach expressed, that inertia depends upon the mutual action of bodies, is contained, to a first approximation, in the equations of the theory of relativity; it follows from those equations that inertia depends, at least in part, upon mutual actions between masses. But this idea of Mach's corresponds only to a finite universe, bounded in space, and not to a quasi-Euclidean, infinite universe. From the standpoint of epistomology it is more satisfying to have the mechanical properties of space completely determined by matter, and this is the case only in a closed universe.
3. An infinite universe is possible only if the mean density of matter in the universe vanishes. Although such an assumption is logically possible, it is less probable than the assumption that there is a finite mean density of matter in the universe.
So this tells us that we need to understand inertia before we really can say much about the limits of space and our universe, which brings us to the Sciama's Ph.D. dissertation. It was entitled 'On the Origin of Inertia'. The abstract tells us that:
As Einstein himself was the first to point out, general relativity does not fully account for inertia. Thus a new theory of gravitation is needed.
The abstract goes on to refer to:
a precise theory, according to which local observations of inertia suffice to specify the large-scale structure of the universe.
So here we see that the scale of the universe, its shape and its boundary form, as well as its evolution over time, are matters intimately interwoven with the theory of relativity, but ever dependent upon our understanding of what it is that endows a particle with its inertial property or mass. It is not sufficient to declare that E=Mc2; one must know why it is that E is equal to Mc2!
The Mach principle was deemed as governing inertia back in 1954.
Well, a decade later, I had evolved my own theory of gravitation based on a structured aether and the numbers, meaning those that related to the relationship between G, the constant of gravitation, electron mass and charge etc., came out right only if I assumed that E=Mc2 was attributable to the non-radiation of energy by the accelerated charge. Here I was saying that if you try to accelerate an electric charge by putting it in an electric field, i.e. by causing other charges to act upon it, it will react to resist dissipation of its energy. It will seek to preserve itself - a normal reaction one can surely expect - and that means a reluctance to move spontaneously as it prefers to move in just such a way as to keep its energy in proper order. Here was the the property of inertia and that E=Mc2 formula was derived on that basis, namely that energy is conserved and not shed by radiation. In one single step I had circumnavigated around Einstein's theory and solved the mystery of inertia, but I had no need to even think about the far-off deployment of mass at the outer bounds of the universe and its gravitational interaction with the particle in question.
I visited Cambridge from time to time and one day called in to chat about this with someone in the Department of Applied Mathematics and Theoretical Physics in Silver Street. That 'someone' who gave attention to my request was Sciama. He invited me to join him over his tea break and we talked about my theory. Sciama was courteous but I had scored no success. I left with my ears recollecting his words of advice: "if you write about these matters you should not use the word 'aether'. Yes, there is an 'aether', but we call it 'space-time'"It was another ten years on before, the E=Mc2 proposition of mine, though published in my 1966 book The Theory of Gravitation was finally accepted as a formal peer reviewed paper by the International Journal of Theoretical Physics, 1976b.
So far as the above Einstein conclusions are concerned, by eliminating the Mach principle as an explanation of inertia I had no need to be concerned about whether the Universe was closed or infinite, but I do wonder how Stephen Hawking can theorize on these matters without a proper understanding of the nature of inertia.
I will come now to my own theme concerning the boundary conditions governing the medium that fills space.
Firstly, imagine that someone tells you that the surface of the United States of America is expanding steadily. That either means that it is growing at the edges where it interfaces with the oceans or it is literally stretching outwards everywhere as if it is supported on a elastic base which is expanding. The question one might then ask is how this might affect the division of U.S.A. into its several states. Would there be need to add new states to the areas formed at the boundaries or will all the existing states get progressively larger in the process?
Now, that is not something one needs to worry about, but let me now come to my second point. Imagine instead a block of steel which is progressively extended by welding on more and more steel. As physicists already known and as cosmologists should already know, that body of steel comprises minute 'states' called 'magnetic domains', each one populated by many trillions of iron atoms. Those domains, which have planar boundaries and measure of the order of 100 microns between adjacent planar boundaries, do not expand in proportion to the growing size of that steel block. In fact, new domains are added as the block grows, because those domains are governed by macroscopic physical conditions not deriving from the individual component atoms within the domain. Those conditions are linked to the collective properties of a number of those atoms, determined in conjunction with the electrical properties of the underlying medium, space or aether, however you might choose to term it, but really the capacity of that medium to store field energy.
In short, there is, in every piece of steel, indeed in every ferromagnetic substance, a latent presence of domains which exist in their own right, governed solely by the way in which energy present chooses to deploy itself to suit its optimum lifestyle.
Now, what I am coming to by this argument is the proposition that, on a very large scale, extending throughout outer space, the presence of matter which we see as the 'universe' exists in an all-pervading system of space domains each of which has planar boundaries. This is not really hypothesis, because if you do not accept what I am suggesting then you have grounded yourself as a physicist until you wrestle with and resolve the issue of how it is that the energy density of an electric field in the vacuum medium can possibly have the value as formulated in your textbooks. Yes, you can say that empirical data based on observations here on body Earth are sufficient for your needs, but if you are a theoretical physicist, especially a cosmologist, you cannot build your notions with confidence unless you can deduce that energy density formulation by theory which takes full account of boundary conditions applicable in space, even in outer space!
At this point it may comfort the reader if I quote two paragraphs from a paper on this subject published by the Italian Institute of Physics in 1983. See: 1983k in these Web pages.
Modern physical theory is tending to regard the vacuum medium as having structure somewhat analogous to that of crystalline materials. Thus we see WEISSKOPF [1] discussing quantum electroweak dynamics and asserting that the Higgs field implies that the vacuum has a certain fixed direction in isospace, namely that of the spinor associated with the Higgs field. WEISSKOPF states that the situation is like that of a ferromagnet, in which the direction in real space is determined as long as the energy transfers are smaller than the Curie energy.This, of course, implies an ordered structure of the vacuum medium, a feature discussed at some length by REBBI [2] in an article entitled The Lattice Theory of Quark Confinement. REBBI refers to a 1974 proposal by Wilson that QCD (Quantum Chromodynamics) should be formulated on a cubic lattice, an array that divides space and time into discrete points, but is essentially an approximation to real space-time. The advantage is that this allows calculations to be made that would otherwise be impossible.
The references just introduced are:
[1] V. F. Weisskopf: Physics Today, 69 (1981)
[2] C. Rebbi: Scientific American, v. 248, 36 (1983)
You see! Physicists have a very complicated way of communicating their agony at not being able to fathom what is really going on in space. Given that admission that there is the glimmer of a connection with ferromagnetism, let us move on by keeping our physics in proper perspective.
The planar domains in steel should be your clue, if you venture to theorize about the expansion of the universe and wonder about its outer bounds. Indeed, you should be very careful about how you define your 'universe', because there is that something in space that provides the storehouse for the energy which comes with your material universe and you cannot just ignore its extent and the simple question of whether it also 'expands' with your universe. If you believe in Einstein's theory then you really have to weigh how that four-space system shares that expansion.
Where Stephen Hawking's 'cone-shaped' universe expanding from a point and destined to go on a never-ending trek all the way to infinity, fits into the picture of reality, you must judge for yourself. My case is that space contains planar boundaries and I do not see them as part of a universal expansion. Do not be deceived by the conical sections I shall introduce below to develop the mathematical analysis. I am merely sectionalizing space into segments defined by spherical angles to perform elemental analysis before merging the results to apply to space as a whole.
Note that the task is to explain how energy is stored in space. To store energy in a linear system you need to do work against a force arising from the distortion of whatever it is that fills that space. By linear system I mean one with a linear force rate. Twice the force implies four times the energy stored. This is the essential ingredient of simple harmonic motion, and related oscillations, as well as the property of electric and magnetic fields. So, space must contain something that has two parts which it tries to keep together but which we separate slightly by storing energy.
The obvious assumption then is that we are talking here about electric charge, which comes in positive and negative forms that attract one another, but which can, at least in a macroscopic sense, compensate one another if not displaced by storing energy. The question then is how boundaries can form within such a system to create something analogous to those domains. To answer this we need to introduce an asymmetrical property. There is something special about negative charge compared with positive charge in what we see as our local space, but that feature inverts as between the two charge polarities in an adjacent region of space, thereby giving scope for defining a boundary between two types of vacuum medium, shall we say 'space' and 'anti-space'?
Speculate if you will about how this can be and be guided by a clue I now offer. Maybe positive and electric charge are really only states of an oscillating system. All positive charges share the same oscillation phase and all negative charges share the same phase of oscillation but the latter are in anti-phase with the former. Yes, that involves instantaneous action at a distance, but we are talking here about electric charges fields and not propagating disturbances characterized by electric and magnetic field effects. Remember that quantum theory requires action at a distance to be effective in the Coulomb gauge.
What then is that asymmetry? I will illustrate what I have in mind. Referring to the following figure, suppose that in one domain of space there are negative charges which are depicted in white and that these are set in a a continuum of positive charge which is depicted by a black background. The amount of charge of the two opposite polarities is the same in any domain. It is just that one form is concentrated in a kind of particle form, whereas the other is spread thinly as a uniformly dispersed charge form. Then, in an adjacent space domain, these charge forms are reversed, the negative charge being the background continuum and the positive charge having the particle-like form.

There will be a planar boundary between the two space domains. Now you may ask why this is important and the answer to that emerges once we consider the effects of actions which displace those charged particles from their positions of equilibrium. This is an action which stores energy, the physical basis for electric field energy as stored in the vacuum.
We shall suppose that all those charged particles in a given region of space are displaced in unison when an electric field is present and this means that they retain their relative positions. The force we are considering is the force on each such displaced charge that arises from the electric interaction with that background continuum in the local space domain.
We will define an area A subtended by a cone, the apex of which is at point P and which has a spherical angle S. See the following figure.
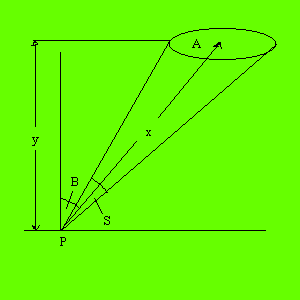
Our object is to determine the force acting on a charge at P and attributable to a planar slice of the background charge continuum distant y from P. That planar slice is parallel with the space domain boundaries of the domain region in which P is located. See the next figure, where the boundaries are those separating the local (green) domain and the adjacent (black) domains.

Let q denote the charge density of the background continuum and assign the charge at P a unit value. Then the charge in the section denoted by the area A is qA(dy), where dy is the elemental thickness of the planar slice of the continuum as shown. The electrostatic force acting on the charge at P will be an attractive force pulling the charge towards the planar slice. By the inverse square force law it will be of value qA(dy)/x2 directed at an angle B to the line drawn at right angles to the planar form. Accordingly, the component force pulling the charge in the normal direction will be given by:
Now, AcosB is x2 times the solid angle S. It follows therefore that the force expression in equation (1) can be written simply as:
To work out the overall effect of displacing the charge at P towards the planar boundary, we simply note that we are displacing it from a position of equilibrium and so a step through a distance y will reduce the action of a slice of continuum of thickness y in front of the charge and enhance the action of a corresponding slice of continuum behind the slice. The former involves a reduction of the attractive force by 2(pi)qy and the latter corresponds to a restraining force of 2(pi)qy. Together these amount to a restoring force acting on the unit charge of 4(pi)q per unit charge per unit distance of displacement.
You can then work out why it is that, in cgs units as used in the early days of field theory in physics, the energy stored in unit volume of space by electrical displacent within the vacuum state is 1/8(pi) times the square of the electric field strength. The force is subject to a linear rate and half the resulting force times the distance through which it is displaced gives the energy involved.
We therefore know, or should know, that there is something in space that is affected by that electric field condition so as to be able to store energy. To comply with what is observed I am saying that there are two opposite kinds of electric charge that are pulled apart just a little in storing that field energy. Now, I could say that, given that the applied electric field is uniform over the region where we are interested in energy stored, that small displacement of charge must, in effect, amount to the development of a planar slice of electric charge. In that case, it seems irrelevant to worry about the ultimate boundary conditions governing space; everything that is affected is local to the test region. Indeed, when I first developed my theory that was my way of looking at things. However, then, sometime around 1966-1967, my employer IBM suggested that I visit Professor L. H. Thomas at Columbia University in New York to see what he thought of my aether theory and its gravitational aspects. He urged me to consider and take full account of boundary effects, as these could affect my conclusions.
Now, for my part, since I believe in the reality of the aether as containing a uniform continuum of one charge polarity populated by a lattice-like array of identical discrete charges, which, being crystal-like itself, can nucleate its structure on material crystals, I regard the planar boundary state as an implicit property of the aether. If scientists are unwilling to accept the real aether of this form then they need to find their own way of giving physical form to the field energy storage process. My theory, however, implies conditions governing the gravitational action, conditions which demand harmonious motion such as stem from that linear restoring force rate deduced above.
In order to derive the law of gravitation from electrodynamic analysis and go on from there to derive the value of G, the constant of gravitation, neither of which are possible based on Einstein's theory, one has to accept that harmony of motion by charge displaced in unison in that non-emptiness of outer space. The seat of the gravitational action is the system that is in dynamic balance with that charge motion. This is all explained elsewhere in these Web pages by reference to my published work of academic record. However, the planar boundary problem that we are discussing is part of the story.
Possibly Professor Thomas had Mach's Principle in mind when he stressed the boundary factor. That principle suggests that the mass of a particle here on Earth depends upon its gravitational interaction with all matter extending to the bounds of the universe, but I do not subscribe to that belief. I do believe that the rhythm of the aether charge motion has to be preserved within each and every space domain in order for gravitation to be a universal force within that domain, but I suspect that mass on one side of a planar domain boundary will not interact in the normal gravitational sense with mass in an adjacent boundary.
For that reason I submit that one needs to study the major geological upheavals and reversals of the Earth's magnetism that occur from time time, because that signals an event lasting a matter of seconds when body Earth, moving at 400 km/s, relative to the cosmic background, is carried through a space domain wall. We measure that motion by assessing the thermal condition of space through which we are travelling at cosmic speed. Though isotropic, its anistotropy, as we see it, tells us how fast we are moving. The temperature measured tells us the strength of the gravitational potential of matter confined within our local space domain. You see, the energy released by the gravitational interaction between matter and those aether charges in space has nowhere to go other than into a state of thermal agitation of the aether charge, a motion superimposed upon its rhythmic oscillations. So we can relate the individual mass of each of those aether charges with its temperature and the gravitational potential at the point in space where it sits. The 2.7 K temperature background of space can be calculated by such theory, if the gravitational action of the solar system approximates that of the local gravitational potential. See my paper 'The Determination of Absolute Gravitational Potential', 1983d.
Such evidence compells me to believe that there are those planar boundaries in space, even though they exist with a separation distance that can be 100 or so light years, meaning that body Earth experiences those major upheavals at intervals that can be as long as a few hundred thousand years. See the 'Feedback' comment elsewhere in these Web pages: Feedback Note 02.
Now, before I leave this subject and leave you to choose whether to believe Stephen Hawking or believe what I am saying here, I will invite review of the Appendix added below. It is quoted from the published paper: 1983k. I just wish to emphasize the point that if the boundaries of space were spherical in form, then the harmonious oscillation property would not have the form needed to explain the known energy density property of electric field theory, and if the boundaries of the universe were conical, as Hawking believes, I doubt if one could ever derive a theoretical value for the constant of gravitation. There seems no point in working out the ultimate shape of the universe, if one cannot explain the existing property of gravitation here on Earth!
If the elements of this vacuum structure are in a state of stable equilibrium and comprise electric charge, then, in order to satisfy Earnshaw's law, they must pervade a charged electrical continuum of opposite polarity. The latter is necessary to assure stability by providing a restoring action upon displacement. Accordingly, the only feasible model for a vacuum state having structure is one for which the discrete charges e of the same polarity interact to form a lattice within a continuum of opposite charge density q. It seems logical that the charges e are of equal magnitude and that q is uniform over a local region of space.Then, by simple analysis, one may show that if a lattice parameter d is written to satisfy:
e = qd3 .... (1) the electrically-neutral state of the vacuum implies that each charge e takes up a space volume d3. With a uniform electric field of intensity E applied we find that the charges e will all be displaced in unison to satisfy:
Ee = ky ..... (2) where k is a constant restoring force rate and y is displacement. In effect, the whole lattice is displaced relative to the background continuum.The energy density stored by this displacement is:
W = ky2/2d3 ...... (3) or, from (2):
W = (Ee)2/2kd3 .... (4) and, as this is E2/8π, for a vacuum of unit permitttivity, we find that k is given by:
k = 4πe2/d3 = 4πqe .....(5) from (1), thereby justifying the statement that it is constant.The use of this restoring force rate is fundamental to classical theory and it might seem somewhat elementary to have derived it from the electrically neutral vacuum model under discussion. However, the problem emerges upon analysis of radial displacement of a charge q within an arbitarily spherical bounded system. At a distance R from the centre of a sphere of continuum charge the total continuum charge acting on e is 4πqR3/3. The electric field is 4πqR/3, where R is now a vector.
For any displacement x, as shown in the figure below, a charge e at P will be subject to a restoring force which is the expression 4πq/3 times the vector difference between two radius vectors R and R'. This is simply 4πqx/3. It follows that if e is part of a rigid lattice which is displaced as a whole by the distance x within the bounding spherical continuum, then the lattice is subject to restoring forces which are only one third of those expected from equation (5).
