return to homepage
return to updates
A Call to
Astronomers

by Miles Mathis
Astronomers don’t field many fundamental theoretical predictions on solar system bodies these days. This is not because they are uninterested in nearby objects, but because almost no one is making such fundamental predictions. The only really active theory is string theory, and it doesn’t offer much in the way of observable data, especially concerning near-Earth objects. A few esoteric predictions are still made occasionally outside string theory, but the kind of basic predictions that scientists used to make are not as much a part of astronomy as they used to be. As recently as the time of Einstein, basic celestial prediction was crucial. His predictions for two of the bodies closest to us, Mercury and the Sun, were critical parts of General Relativity, and of its acceptance by science. But this sort of revolutionary physics is thought to be over. It is thought that our knowledge—especially of nearby astronomical objects—is so nearly complete that revolutionary physics is a thing of the past.
I will show that this assumption is premature. In this paper I will make a fundamental theoretical prediction that is easily confirmable. It does not concern black holes or quasars or other mysterious objects. It concerns the largest planet in our own solar system, Jupiter.
In my paper on GR I showed that I could easily achieve the same number that Einstein achieved for the bending of starlight by the Sun, without using Gaussian fields or the tensor calculus. I used only the first postulate of General Relativity—that of equivalence. In four lines of high-school algebra I derived the number 1.7, using only two given numbers: g (the gravitational acceleration of the Earth) and AU (the distance to the Sun). Having such success, I then applied my method to Jupiter. Einstein had predicted .02 seconds of arc for the bending of starlight by Jupiter, but I found a much larger number. Realizing that this larger number would be seen rather easily by our modern telescopes, I decided to apply directly to contemporary astronomers to put my prediction to the test.
There are several reasons this should be of interest to science, even if science has no particular interest in me or my theory. The primary reason is that General Relativity was hurt by the discovery that Eddington had followed a rather sloppy procedure. It was hurt further by the inability of later astronomers to offer conclusive data that supported Einstein. To this day, the bending of starlight by the Sun is an unfinished matter. General Relativity has been verified by other means, it is true. But astronomers should still like to verify it by their own methods, I would imagine. If they could do this conclusively by looking at Jupiter, I should think they would jump at the chance.
A secondary reason is that I provide more than a prediction in this paper. I provide a procedure that shows precisely why Einstein’s prediction has not been verifiable. His prediction for the Sun matches my own, so that I will not claim that his prediction has not been verified because it is false. I will show that his prediction is not verifiable because he failed to advise astronomers that different positions on Earth would give them different data. I provide diagrams that show a simple reason for this variance, and that predict the exact variance for different locations, both for Jupiter and the Sun. This should allow astronomers to verify Einstein’s prediction for the Sun. At the same time it will allow them to verify (or falsify) his prediction for Jupiter, in the same way.
A final reason is that my prediction for Jupiter is so large and so different from Einstein’s that there can be no overlap in the data. It will be quite easy to falsify my prediction, which would be one more feather in Einstein’s cap. I have no reputation, so that overcoming my prediction might be seen as small consequence to Einstein. But at this point in history, Einstein is frankly in need of a new conquest, no matter how small. A new astronomical confirmation of General Relativity, using a planet in our own solar system, would benefit physics greatly. It would also make some astronomer quite famous. We would have a new Eddington.
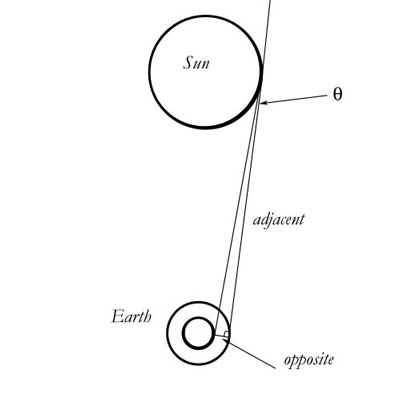
Here, then, is my prediction. We will start with this diagram that I used to calculate the bending of starlight by the Sun. It applies in the same way to Jupiter. You can see that the distance from Earth to Jupiter is one of the primary variables of the math, so my prediction must vary as that distance varies. To begin with, I will calculate in the situation that the Earth is nearest Jupiter in their orbits. I will take 4.2AU as this distance. At this distance, Jupiter is 2095.8 light seconds away from earth.
There is a single postulate that underlies both this diagram and the math that will follow. That postulate is provided by Einstein himself, and it is one of the first postulates of General Relativity. It is the famous postulate of Equivalence. Einstein showed the mathematical and conceptual equivalence of gravity and acceleration, using an elevator car in space as his thought problem. Equivalence in that situation meant that a person in the car could notice no difference if we reversed the acceleration vector. We could give the car an actual acceleration up or we could put him and the car in a gravitational field, where the acceleration vector pointed down. Generalized, equivalence means that a reversal of the main acceleration vector of a field will imply no mathematical differences.
Now, I have theorized that we can apply the postulate of equivalence to the field of the Earth. If we reverse the direction of the vector g, in order to mathematically flatten out the gravitational field of the Earth, then this has the physical outcome of making the surface of the Earth accelerate in all directions (that is, spherically). Einstein did not follow this mathematical course for this very reason. He did not like to imagine the Earth expanding. But we do not have to believe that this acceleration is real. We can accept it only as a mathematical concept, one that simplifies our math. We can therefore turn it back around after we do the math, if we like, bringing us back to Standard Theory.
In this way my operation is much like Minkowski's operation when he produced his mathematical fields. He was not concerned with whether his fields were physically real or not. They were a mathematical convenience. Minkowski was never concerned with proving or disproving that time physically travelled at a right angle to x, y , z. He proposed assigning t to the hyperbolic mainly because it made his math symmetrical, and this symmetry allowed him to express Relativity in what he thought was an elegant manner.
Likewise, reversing the vector g may be seen as a mathematical convenience and nothing more. But if we allow ourselves to reverse the vector, then after a time t, the surface of the Earth will have moved a distance s in all directions.
s = gt2/2
t = time for light to travel from the tangent of Jupiter to the Earth
= light time from Jupiter to Earth + light time of the radius of Jupiter
s = (9.8 m/s2)(2095.8 + .24s)2]/2
s = 21,530,000m
tanθ = opposite/adjacent
tanθ = 2.153 x 107m/6.29 x 1011m
θ = 7.06 seconds of arc
Using this diagram and math, I found 1.686 seconds of arc for the Sun. You can see that I have found a much larger number for Jupiter simply because Jupiter is further away. This means that we should be able to see way around Jupiter, provided that we take our picture off the right edge of Jupiter from the right side of the Earth (as seen in this diagram). If we take a picture off the left edge of Jupiter from the same telescope, we will find many stars obscured that should not be. An additional 7 seconds of arc (roughly—the tangent there will not be exactly 180 degrees from our first tangent, but close enough) should be obscured by the left edge.
Of course, the number 7 seconds of arc applies only when the Earth is closest to Jupiter. Even greater apparent bending could be achieved by placing the Earth at different positions relative to Jupiter. Let us put the Earth sideways to the Sun, for instance, and do the math.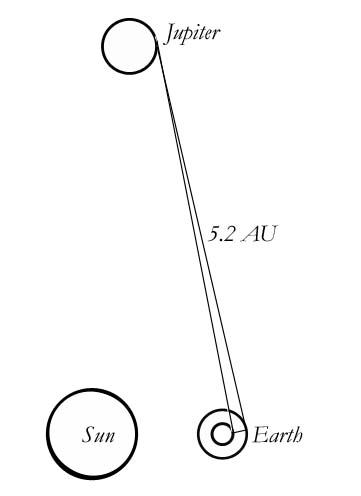
tanθ = 3.3 x 107m/7.80071 x 1011m
θ = 8.73 seconds of arc
Positions of the Earth much further than this away from Jupiter will not be experimentally feasible, since Jupiter will be up only during the day.
From my mathematical diagrams, it is clear that viewings of Jupiter (or the solar eclipse) must be done as close to the horizon as possible. Only in this way can the astronomer be sure that he is positioned as far to the edge of the earth, relative to Jupiter, as possible. I am aware that this brings other factors into play, but it is unavoidable.
As one final peculiarity of the experiment, notice that the scientist will actually be studying the top and bottom edges of Jupiter, as he sees it on the horizon, not the left and right edges. I have positioned the telescope on the far right edge of the earth relative to Jupiter, in my diagram. But if we imagine a little man standing on the earth at that position, his head must be pointing due right also. If we rotate the whole diagram so that he is standing up like we are, then he will be studying the top and bottom edges of Jupiter as he sees it. The top edge will give him the number for θ above, allowing him to see around Jupiter a bit; the bottom edge will obscure stars that he expects to see.
The experimental difficulties of this viewing would make it unlikely to yield results, except that there is now such a huge difference from edge to edge. Also notice that it can be measured from the same location—we don’t need to travel or to compare data from different telescopes. And it doesn’t even require great precision. The clumsiest measurements will show a variation from side to side, if it exists as I predict. Seven or eight seconds of arc can be seen by medium sized telescopes, even on the horizon, provided the location and atmospheric conditions are optimal. The only thing that takes special care is in choosing the location. A telescope positioned near the center line of the Earth, relative to Jupiter, will show almost no aberration and therefore almost no variation.
Now that we have explored this problem both mathematically and theoretically, we are in a position to see why Einstein’s prediction has never been completely verified by data from eclipses. After Einstein’s fame had already been achieved by Eddington, Eddington’s method was called into question. It was found that he had pushed his data strongly in the direction of Einstein. New experiments in the 20’s and 30’s failed to verify the number 1.7, and all experiments since have been inconclusive. It is now easy to see why. Given the current explanation of aberration, scientists have not expected that the position on earth of their telescopes was important, except as regards normal considerations like clarity. They never factored in what I have shown must be the main consideration, if my theory is true. Different positions on earth must give us radically different numbers, from +θ on one edge to zero in the middle to –θ on the other edge. The variation in Eddington’s numbers at the two different locations can now be explained as (mainly) a difference in longitude. Brazil and the west coast of Africa are almost 30o apart, and should not be expected to yield the same bending.
I repeat, if an astronomer positions his or her telescope near the center line of the Earth (relative to Jupiter in these diagrams) then he or she will find no bending, according to my theory. Furthermore, the astronomer should not expect to find the predicted bending on stars sideways to Jupiter. The predicted bending and obscuring will occur only for stars immediately above and below Jupiter, above and below having the normal meaning for an astronomer standing on the Earth and looking at Jupiter near the horizon.
If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.