return to homepage WHY IS THE SKY BLUE?
return to updates

for the world is hollow and I have touched the sky
Abstract: I will show that the current explanation is wrong. The color blue is not caused by scattering by molecules in the atmosphere, it is caused by interaction with the Earth's charge field.
In researching this topic, I was surprised to find two different answers from two of the top mouthpieces of current physics. The top answer on a Yahoo search is by Philip Gibbs at the University of California, Riverside1. It is that blue light is bent more than other colors. Gibbs shows us this illustration:
If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.
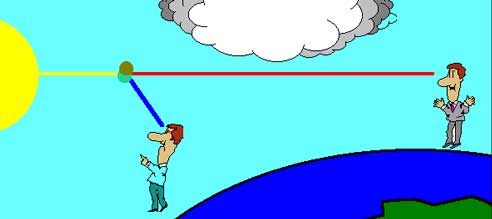
Problem there is that we can move the Sun a few degrees and switch that effect. Just take the Sun above the first guy and he sees an unbent blue ray. The other guy sees a bent red ray. Light must be coming from all directions, not just the direction of the Sun, so this illustration is more than useless, it is misleading.
Gibbs then shows it is air molecules, not dust, that do the bending, and he tells us that Einstein calculated in 1911 the scattering by molecules. These equations of Einstein are said to be “in agreement with experiment.” We are told, “the electromagnetic field of the light waves induces electric dipole moments in the molecules.” We aren't told how it does that.
Gibbs then asks the million dollar question: “Why not violet?” If short wavelengths are bent more than long, then violet should be bent even more than blue, and the sky should be violet. He says it is due to the cones in our eyes. He shows the figure here
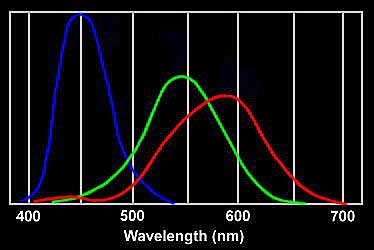
and says that violet light stimulates red as well, making us see blue. We will come back to that answer and diagram in a moment.
The second top answer is from NASA, and is for kids. I would leave it be for that reason, except that the third2 and fifth and sixth top answers are strictly the same as this one. All these answers are glosses of the only slightly longer and fuller answer at Wikipedia, which is fourth only because it is titled “Diffuse Sky Radiation.” That answer is that the blue sky is caused by Rayleigh scattering from molecules. Molecules scatter short wavelengths more than long wavelengths.
The Wiki answer is the fullest I found on the web, but even it is short, without the usual attempts to misdirect with large amounts of math or complex theory. We are sent to the page for Rayleigh scattering, but we get one equation and then the whole theory: “the shorter wavelength of blue light will scatter more than the longer wavelengths of green and especially red light, giving the sky a blue appearance.” Wiki doesn't even bother to spin some yarn to explain “why not violet.”
So let's look at this theory, such as it is. We already have two theories, although they seem to be similar or equivalent at a first glance. Gibbs' theory is that since red light is bent less, it never reaches the ground except near sunset. We get this impression from his illustration, although he never states it explicitly. It is slightly preferable to the scattering hypothesis and the scattering illustration, since it begins to address a smart kid's question: “If red is scattered less, wouldn't it just reach the ground directly, with more intensity?” So Gibbs draws bending instead of scattering. You see Gibbs has a few dormant scruples left, which makes him try to tweek the horrible answer he has been given from above. He also tries to answer the “why not violet?” question, although he clearly isn't up to it. His own figure, from eyeball physiology, shows in technicolor detail that the green receptors are also stimulated at violet wavelengths, so why talk about the red and ignore the green? He isn't making any sense. Even if the red were stimulated while the green were not, you still can't build blue from violet plus red, at least not according to the current model. Since the retina is not white, you can't stack these red and violet responses to get blue. That would require red canceling the red in violet. But if the eye is seeing both red and violet, that will make the violet more red, not less red. You can't make violet into blue by adding red in paint mixing, CMY, or RGB. The current model is an RGB model, and when crossing violet light and red light on a white background, you don't get blue. In RGB, you cross red and blue to get magenta. Just the opposite of his implication.
But all this is moot, since his figure shows a green response, too. We need to mix red, green, and violet, to address his logic. In RGB, that should give us something quite near white. In CMY, it would give us something nearer black. His whole explanation of “why not violet” is a wash, and he would have been better to come up with a different dodge.
Gibbs' semi-honesty also comes through in his ability to admit that violet is not lacking in the sky. Some have tried to answer “why not violet?” by claiming that our eyes are not as sensitive to it, or that it is absorbed by the high atmosphere, but Gibbs admits that it must be there, and that we must be sensitive to it, since we see it very well in rainbows.
But back to the main question. Forget violet. Can bending or scattering explain “why not red?” Suppose that atmospheric molecules do scatter blue more or more often than red: does that explain the lack of red in the sky? No. To explain the lack of red, you need to keep red from hitting the ground, so you need to propose that it is bent so much it goes back up, or it is absorbed so much that it never comes down. Neither scattering nor bending does that or can do that, at least not with the current model of light. No smart schoolkid will be fooled by Gibbs replacing scattering with bending, since his question remains: “If red is either scattered or bent less or less often, shouldn't it just have a more direct path to the ground?”
The answer Gibbs is trying to shield or prop up is the scattering answer, which uses Rayleigh scattering as the whole explanation of the blue sky. This is what we get from Wiki:
The strong wavelength dependence of the scattering (~λ-4) means that blue light is scattered much more readily than red light. In the atmosphere, this results in blue wavelengths being scattered to a greater extent than longer (red) wavelengths, and so one sees blue light coming from all regions of the sky.
Notice that Wiki has just interpreted the Rayleigh molecule equation in two different ways. In the first sentence, we are told that the wavelength dependence means that short wavelength light is scattered “more readily.” In the second sentence, we are told that short wavelengths are scattered “to a greater extent.” This leads us to ask, “Are the short wavelengths supposed to be scattered more often, or at a greater angle?” If we study the Rayleigh equation carefully, we find the answer is the angle, not the probability of being scattered.
I = I08π4α2(1 + cos2θ)/λ4R2
As you see, we have a representation of the angle, but no representation of the probability of being scattered. The constant α is the polarizability of the molecule, so it cannot vary with the wavelength of the light. The Rayleigh equation gives us an intensity of the scattered light, given an incoming wavelength. In other words, the Rayleigh equation requires the incoming light to be of one wavelength. We can only insert one wavelength into that equation at a time, as you see. We let red light hit a molecule, for example, and the equation tells us how intense the scattered white light will be. This is because the scattering turns the red incoming light into all colors (or, rather, no colors). That is what scattering means. That is why the sky is bright instead of dark. If we subtracted out the color problem, we would explain the whiteness of the sky as due to scattering. That is why space is dark: no molecules are scattering, creating the whiteness.
We can see this from Wiki, on the page for scattering.

Scattering changes or destroys the original wave, as you see. You cannot scatter a wave and keep the wave at the same time. That is having your blue and eating it, too. Despite this, current theory assumes that a wavelength that is blue is scattered, remaining the same blue wavelength; that if anything it gains intensity from the Rayleigh equation; and that it is then reflected down (but not rescattered) by other molecules. Question: why do the first molecules scatter it and the second molecules just reflect it down? How do molecules know when to scatter and when to reflect? Amazing, really, that air molecules can redirect blue so many times without affecting it, but that air molecules cannot do that with red (except at sunset, of course).
To see how dishonest all this is, we can study this figure at Wikipedia:

Figure showing the more intense scattering of
blue light by the atmosphere relative to red light
They have created a figure to show what they want to show, although the Rayleigh equation doesn't show it. The figure shows more scattering of blue as a percentage of incoming light, and just in case you missed it, they make the upper part of the figure sky-blue and tell you one more time the conclusion: “Rayleigh scattering gives the sky its blue color.” They just need a manipulative soundtrack and a nude woman waving a magic wand and the propaganda would be complete. But even the figure contradicts itself, since they feel obligated to cover themselves by giving us a bit of subtext: “figure showing more intense scattering of blue light....” Wait, they just said in the figure's sidebar that the figure showed more scattering as percentage, and now they are saying the figure shows more intense scattering. But, as I just showed you, the two claims are not the same. The equation does indeed show more intense light scattered from incoming blue, but it doesn't show more scattering of blue as a percentage of all incoming light. Our brain is being massaged here.
If we apply the Rayleigh equation to all the different wavelengths, it is just telling us that our scattered white light will be more intense if we start with short wavelength light instead of long wavelength light. And that is just because short wavelength light has more energy to start with. It has a greater frequency, and therefore, according to the Planck relation, it has more energy. More energy supplies more intensity in scattering.
So, you see, the Rayleigh equation doesn't tell us a damn thing about color. It doesn't tell us that blue light is scattered more often, or even more. It isn't scattered more, it is scattered at a greater angle, and that just tells us that scattered blue light will cause more intense white light.
Current theory assumes that Rayleigh scattering is elastic, since it needs to keep the blue light blue. But there is no proof of that other than the color blue we see. They have no mechanics to explain it or prove it, and since all the math is pushed to fit the data, the math cannot be proof of anything.
You see, Rayleigh scattering is even less understood than Compton scattering7 or Thomson scattering. At least in these two theories of scattering, we have some mention of photons. We have particles acting on eachother: if not colliding, then at least exchanging energies. Beyond that, we have emission and emission fields. But with Rayleigh scattering, we have none of that partial rigor. We just have an equation, derived by slippery means, and then an illogical theory tacked onto it. We are shown a wavelength dependence in the equation, but then the theory and explanation stops. We aren't told how this explains blue reaching the eye but not red. We are told the blue is scattered more than the red; but then what? If the red isn't scattered so much or so often, wouldn't it just continue on down to the ground? And if so, why is it invisible?
Current theory has a huge hole in it, and it becomes very conspicuous in this problem of scattering. We are given an equation for scattering but no explanation of it. Nowhere on the web or in any book could I find a definition of this sort of scattering. What is happening mechanically? No one knows, because they will not define the light as either a wave or a particle. We aren't told if the wave is being scattered or if photons are being scattered. All the math and sentences imply it is the wave, but none of the specifics are ever addressed. The quantum mechanical explanation of scattering is currently the Feynman explanation, using sum-overs. In this explanation, Feynman, like non-quantum explainers, mainly avoids any mechanics. Feynman tells us it is photons that are scattered, but he likes to use his little clocks and vectors8 to solve these problems, and he will not admit that these clocks and vectors track the waves. Clocks are waves. At any rate, this is the nearest we get to a mechanical explanation of photon mechanics, but it is a purposeful misdirection. Feynman and other quantum physicists have told us not to ask mechanical questions, which is convenient for them.
Feynman came closest to being able to solve these problems because he allowed himself a math that represented the photon as both wave and particle at the same time. Before that, we have to go all the way back to Maxwell to find someone giving the photons themselves waves (or spins), and Maxwell only tried that for a couple of years before it gave it up. Before Maxwell, we go back to Newton, who tried to give his corpuscles spins (and spins are waves). Both Newton and Maxwell were shouted down or ignored, and since Feynman died quantum physics has ditched his clock method as too visual and mechanical. But treating the photon as both particle and wave is the only way to solve this problem. I have no use for sum-overs or photons with clocks, but we can solve this problem by giving the photon spin.
To solve, we have to throw out Rayleigh's equations, and Einstein's 1911 calculations as well. Because the blue in the sky is not caused by scattering at all. It isn't caused by light interacting with molecules in the atmosphere, although I suppose that was a good guess until now. It is no longer a good guess because we now know about the charge field. The Earth is recycling a heavy charge field all the time, which means that charge photons are rising in huge numbers every moment. It is charge moving up that is interacting with light coming down.
I have said in other papers that photons don't interact that much, and while that is true, they do interact in situations like this, where two heavy fields are meeting head-on. Even here they don't interact that much, and we know that because if they did interact in proportion to their energies or mass equivalents, we would have show indeed. We would have a million-watt aurora borealis all day long. No, they interact only enough to give us a pretty blue.
This is not to say that incoming light doesn't also interact with ions and molecules. It does. We get lots of effects from these interactions. But that is not what this paper is about. This paper is about the color blue, and the color blue is caused by charge interaction. We should have known that, because if the color blue was caused by ion or molecule interaction, it would vary greatly. The ionic content of the atmosphere varies quite a bit, especially from pole to equator, and yet the color of the sky does not vary. Even the Raleigh equation tells us that, since different ions and molecules should give us different colors. They don't, so it can't be the ions and molecules causing the blue.
Charge also varies, but not as much and not in kind. In other words, you can have different ions and different molecules, but you can't have different charge. Charge is always photons, and charge photons vary in size to only a tiny degree. Or, the average charge photon is always about the same. This is why the sky is always the same value of blue.
The charge field is our first new bit of information. The make-up of light is our second. I have shown in other papers that the wavelength of light is not caused or carried or expressed by the wave front or by a group of photons or by any medium. It is caused by each individual photon. Each photon has a tiny local wavelength, caused by spin about an axis, and this local wavelength is stretched out by the speed of the photon. The orbital velocity of the photon is 1/c, and the linear velocity is obviously c, so the local wavelength is stretched out by c2. That is where the c2 in Einstein's famous equation comes from. This stretched out radius is what we experience as the color.
Given that, we see that the individual red photon is smaller than the individual violet photon, at the quantum level. Because it is smaller, it has a smaller total energy. These photons coming down will meet charge photons going up, and the charge photons are infrared. This means they are even smaller than the red photons. In this particular situation, the more energetic descending photons will be affected the least. That would be blue and violet. The red descending photons will be affected the most, because they are closest in energy to the charge photons coming up. Infrared photons will affect red photons more than blue photons.
But to truly understand this interaction, you have to understand how photons interact. Because they are so small, they are most likely to hit edge-to-edge. Center-to-center hits are extremely unlikely. This means that photons interact with their outer spins. The linear velocity c is unaffected. Because blue and violet photons have a greater spin energy, the spin energy of the charge photons affects them less.
What actually happens is that the charge field damps the energy of every photon it collides with. The photons that miss the charge field simply come down to Earth and combine to white, as they did before. But the photons that collide with the charge field are each damped down a tiny bit by the infrared photon they just hit. So each photon will be reddened a tiny bit. This is because the incoming field and the charge field are in vector opposition: when they collide, the spins will subtract.
It is this damping that causes the field to shift from white to blue. Because the red photons are affected more than the blue ones, more red photons are shifted into infrared than blue are shifted to green, say. If we just look at the visible spectrum, the greatest losses will be at the lower energies. We have a spectrum shift, but not a proportional spectrum shift. We lose more red than we gain. Overall, this is a loss of low energy photons and a gain of high energy, which will shift the spectrum toward blue. What came in as white, we will see as blue.
This is also the explanation for how light can arrive with many different wavelengths. The current model cannot show how incoming white light from the Sun can carry all the wavelengths at once. If a wave hits an air molecule in being scattered, how can the wave be red and yellow and green and blue all at once? A wave must have some structure, by definition, and a wave that was green in one place and red in the next wouldn't have any structure. Light is still drawn as a field wave in modern illustrations (in the rare case that these phenomena are illustrated), and a field wave cannot vary its wavelength all over the place. But if each photon can carry the wave, then it is easy to show how white light can carry various colors. If light is not a field wave, then this question answers itself, by simply evaporating. Photons may sort themselves by wavelength, but the energy of the “wave” is not determined by particle alignment or rowing or fronts or anything else. A single photon can express the wave by itself.
Why not violet? Because, to see a violet sky, it would require that violet has an infinite energy relative to charge. We have found that violet is affected less, but not unaffected. Since red is shifted the most by charge, some small part of the remaining visible light is red, a bit more is yellow, a bit more is green, a bit more is blue, and a bit more is violet. We add them all up, and what do we get? We get blue. We get a spectrum weighted by probability toward violet, but not all the way to violet.
You will say that the current explanation could steal that last part from me and ditch all the hoodoo about red being smaller than violet. Yes, they could and they probably will. But the truth is they have the mechanics all wrong. We have no scattering in this particular explanation. The phenomenon is another charge phenomenon.
We see clear proof I am right if we then ask the standard model why clouds are white. We are told that clouds scatter all the light the same amount via Mie scattering, creating white light. So Mie scattering creates white clouds and Rayleigh scattering creates a blue sky. This despite the fact that the math is basically the same for the two. Both Rayleigh and Mie applied Maxwell's equations to similar problems, only Mie's particles were bigger. In fact, Rayleigh's equations were first applied to spherical particles, same as Mie's. They were then tweeked to account for the fact that air molecules are not spherical or otherwise isotropic. However, this tweeking is not where the wavelength enters the equations. Rayleigh included the wavelength variation even before the equations were tweeked for the anisotropy. Why? Because Rayleigh developed his scattering equations specifically to explain why the sky was blue! He needed a wavelength variation and Mie didn't. The equations were pushed to match data.
You can't have large particles that scatter all light the same and small particles that scatter blue more, unless you show some mechanical reason for that difference. Rayleigh couldn't show that reason and neither has anyone else. No one has claimed it is the anisotropy of air molecules that is the cause, although that is the only apparent difference, other than size. They can't do that because, as I said, the Rayleigh equations show wavelength variation even with isotropic molecules.3 If it is not the anisotropy that causes the wavelength variation, is it the size? We don't know, since the mathematicians won't tell us, but Wiki will admit that, “In contrast to Rayleigh scattering, the Mie solution to the scattering problem is valid for all possible ratios of diameter to wavelength.” This must mean that the Rayleigh solution is a subset of the Mie solution; and that means that one cannot work in a fundamentally different way than the other. And that means that we have no explanation for why Rayleigh scattering is wavelength dependent and Mie scattering isn't. The explanation is that the sky is blue and clouds are white, which is of course circular.
As usual, the theorists try to misdirect us from this realization with a lot of fancy math. We are told that, “Quantum mechanical perturbation theory for two-photon elastic processes provides both a powerful and elegant means (e.g.: Craig and Thirunamachandran, 1984)”3 for deriving the Rayleigh equation. But if you honestly believe that a QM perturbation solution will be elegant, you are not paying attention. I suggest you study some QM perturbation solutions and get back to me. What would be truly elegant would be a simple mechanical solution, but QM was forbidden from giving you that by the Copenhagen interpretation, back in the 1920's. Instead, they pile more math on your head to try to keep you from asking for sense. Nowhere in this perturbation solution do they address my question here, as to what is causing the wavelength dependence. The perturbation solution is not more mechanical, it is very much less mechanical. More math is always used to cover less mechanics.
We also get sentences like this: “The polarizability of a molecule will depend on its orientation relative to the direction of the incident light and, in general, is a tensor of rank 2.”3 Yes, but that is just misdirection. I don't give a flying squirrel what rank the tensor is, I want to know what the polarizability of the molecule has to do with its ability to sort wavelengths. Keep your math in your hat, but tell me how a small sphere or non-sphere can sort wavelengths while a large sphere cannot. Until you can do that, I am going to assume all your math is just used to create the answer you need.
To show this pushing of math to force a solution, we may look closer at this page I have been quoting from the University of California at Irving. This author, Chris McLinden, develops the Rayleigh equation as “a limiting case of Mie scattering.”4 But since Mie scattering creates white light, he cannot do this without cheating. He imports equations from his Mie page to develop a Rayleigh matrix, and then says, “and from equation (2.39), the Rayleigh scattering cross-section is...” And we get the Rayleigh equation, complete with the wavelength in the denominator. Unfortunately, there is no equation 2.39 on his website. We assume it is a typo for 3.39, but neither equation 3.39 nor any of the equations around it have any wavelength variables in them. How could they, since equation 3.39 is on his Mie scattering page: Mie scattering is not wavelength dependent! Where did the wavelength come from? We cannot dismiss this as just an unimportant oversight or recent typo, since this is a university website that has been up for over a decade, and that scores number five on a websearch for “Mie scattering.”
At number five on a search for “Rayleigh scattering”, we pull up a website by Philip Laven5 that clarifies this a bit. There we find that the scattering angle is proportional to x3, and that x = 2πr/λ. But r here is the radius of the scattering sphere. So, again, we have to ask by what mechanics a small sphere shows a dependence between r and λ, and a larger sphere does not. And, again, Mr. Laven has nothing to say about it. He has more fancy graphs than Wikipedia itself, but no more mechanics. Like Wiki, he has zero mechanics.
Notice that I can explain white clouds without contradicting myself. I have said that all scattering creates white light, and clouds simply scatter more. Therefore they are whiter. Because the particles in clouds are large molecules (water vapor mostly), neither the blue light nor the red light can dodge them. All the wavelengths are scattered, destroying the blue. I will be told that clouds tend to go to grey, but those are shadows. The greys are always on the dark sides of clouds, when clouds are dense enough to scatter most light up or sideways. The grey is not color, it is relative darkness.
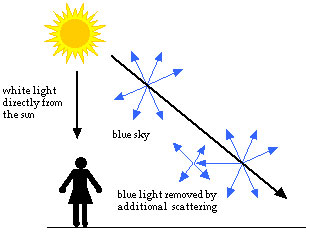
Current theory is also contradictory in its explanation of why the Sun appears yellow. Wiki tells us:
Conversely, glancing toward the sun, the colors that were not scattered away—the longer wavelengths such as red and yellow light—are visible, giving the sun itself a slightly yellowish hue.
So we see yellow because it is not scattered, and blue because it is? That doesn't make any sense at all. Current theory tells us that the blue is coming to us indirectly, and the yellow is coming directly, but we already know that isn't how light works. Scattered light coming indirectly—from all directions—is seen as white light. This is because as light is scattered, its energy is affected by the angle of scattering. Molecules scatter photons at any number of different angles, and blue photons that are scattered are no longer seen as blue. You can't scatter light in all directions and then claim it is still blue. I have already shown why we see blue, and it isn't because blue light is scattered, staying blue.
Just think of it this way: assume current theory is correct, and work back from your eye. Say you are receiving blue from all parts of the sky, except from the direction of the Sun. That means you are receiving blue from all different angles. Go up into the sky, to one molecule that is scattering the light to you. There is an angle at that molecule between you and the Sun. The molecule supposedly deflected the blue photon from the Sun to you, at that angle. Now go to all the other molecules that are doing the same thing. The angle is different for each one. How can the angle be any possible angle, and the light stay blue? According to this theory, any angle of scattering is blue, and no angle of scattering is red. Impossible.
We can see other big problems with current theory. Current theory tells us that yellow light from the Sun is not scattered, and that is why we see a yellow Sun. But in the next sentence, the same theory tells us that in space, the Sun is white. Well, if the light from the Sun is actually white, and the light we see in the line of the Sun is unscattered, then we should see the Sun as white here on Earth, too. They just told us the light was coming directly to our eye, and was not scattered. If it isn't scattered, why is it changed? Why isn't it white? They would have to answer that the violet, blue, green, and red is scattered, leaving only the yellow unscattered. But in that case they have contradicted themselves. In the line of the Sun, we don't see blue because it is scattered away. But everywhere else, we do see blue because it is scattered away. In that case, we have to ask again, “Do we see color because it scattered, or because it is unscattered? You have to make up your mind. You can't have it both ways.” According to current theory, if we see blue because it is scattered, then we should see yellow in the direction of the Sun because it is also scattered. But if that were true, we would have to ask why molecules in line with the Sun act differently. Why do molecules in the line of the Sun scatter yellow? Or, going back to the previous theory, why would molecules in the line of the Sun scatter everything except yellow? It has to be one or the other, but both are illogical. Molecules in the line of the Sun cannot act differently than molecules in other parts of the sky.
The current answer is that molecules in the line of the Sun scatter shorter wavelengths, just like everywhere else, but that is a fudge, since the Sun only looks yellow: it does not look green or red. If the sky scatters blue, then the Sun should look red, green, and yellow. Current theory tries to average these three into yellow, but according to current theory, neither light nor the eye works that way. If we are seeing red, green, and yellow from a source, we will not average that into yellow, we will combine it into brown or grey. Yes, if we are receiving much more yellow than green and red, we will see a greyed out yellow, but that is not the situation with the Sun. The Sun we see is supposed to be white minus violet, and white minus violet is not yellow.
In other words, my average worked above, since I was not averaging three equal parts. I was averaging large amounts of blue and violet with much smaller amounts of green, yellow, and red. This gave me an average of a slightly greyed out blue. But the current model is trying to average equal parts of red, yellow and green to get yellow. Even if they steal my averaging method, they still can't get yellow from that. They get brown or grey. And, besides, they can't use my averaging method, because their theory is not a color averaging theory. Their theory is a physiological RGB theory, where you would cross red and green to get yellow. If you add yellow to that, you get a double saturated yellow. They should predict a very yellow Sun, not a pale yellow Sun.
The reason we see a Sun that appears slightly yellow is mainly because it is next to a blue sky. It is an optical illusion. It is related to this optical illusion called “moth eggs”. 
The “eggs” are actually white, but appear yellow against this background of blue and green. You will say that the sky is not green, but I have shown that it contains some unscattered green, just as this illusion does. This is why the Sun looks yellower in the summer: the green of the trees and landscape reflects off the sky, adding green to the background the eye sees. On a background of green and blue, white will appear pale yellow.
Another reason we think the Sun is yellower than it is concerns the afterimage. We can only glance at the Sun for a split second. If you try it you will see that the yellow is in the afterimage, burned in the retina around the Sun. You don't really see a yellow Sun, you see a yellow afterimage. If you block the circle of the Sun and just look at the sky around the Sun, you will see that the yellow is mostly a myth. We are mistaking bright warm white for yellow. The white looks warm because it is surrounded by cool blue. Even the yellow in the afterimage is false, since it is also caused by the original blue response. It is the retina's response to very white light on a blue background. The retina always tries to supply the contrary color, for reasons still unknown; and the brighter the light, the more the retina supplies the contrary. The yellow circle is just this response to blue: that is why it is only on the edge of the Sun, where the blue meets the white.
Then why is the sky red at sunset? If the current model is upside down, then they must be wrong about that, too. Yes, they are. They say that the blue is scattered less then, but it is actually scattered more. The dust particles that do the scattering at sunset are bigger than atmospheric molecules, so the violet and blue light can't dodge it as well. The violet light is therefore scattered more than the red, leaving a red result.
The current explanation is that red at sunset is caused by particles at low altitudes, from dust, pollution, or other causes. Unfortunately, Rayleigh scattering (and Tyndall scattering, which Gibbs mentions on his page) is not different for larger particles. The Rayleigh equation for particles larger than molecules also has the wavelength variable in the denominator, which means dust particles would not scatter red preferentially over blue, according to current theory. Given current theory, dust should also scatter blue more or more often, because λ4 is in the denominator. These people do not seem to be able to read an equation: they think they can push it any way they like, scattering blue in one case and red in another.
But they are correct that the angle to the Sun is important. At sunrise and sunset, we see red due to reflection from clouds and dust, not scattering. That is, we see a single bounce, at a specific angle. To see red, our eye has to be at a very specific angle to the cloud or dust. Even some editors at Wiki seem to understand this, since at the Tyndall Effect page, we find
The term "Tyndall effect" is sometimes applied to light scattering by macroscopic particles such as dust in the air. However, this phenomenon is more like reflection, not scattering, as the macroscopic particles become clearly visible in the process.
There it is, Wiki contradicts Gibbs, and actually gets something right. We know that the red must be caused by reflection, because we get red in the area of sky opposite the Sun as well as the area toward the Sun. Clouds at the right angle can reflect red back to us.
You will say, “If red is scattered more by the Rayleigh equation, then it should be scattered a lot by dust and the dense atmosphere at low altitudes. So it should be scattered massively in this situation.” Yes, and it is. I didn't say that red wasn't scattered at sunset. I said that the red we see isn't caused by this scattering. The phenomenon of red sunsets is not caused by scattering. The red we don't see is caused by scattering. The red we do see is unscattered light (of any original wavelength) that is reflected to us at the right angle.
To read more about Rayleigh scattering and to see more corrections to it, you may now read my newest paper called The Brightness of the Sky. There I explain the brightness as well as the blue.
1http://math.ucr.edu/home/baez/physics/General/BlueSky/blue_sky.html
2http://www.sciencemadesimple.com/sky_blue.html
3http://www.ess.uci.edu/~cmclinden/link/xx/node21.html
4http://www.ess.uci.edu/~cmclinden/link/xx/node20.html
5http://www.philiplaven.com/p8b.html
6 Mathis, Miles. Planck's Constant and Quantization. 2008.
7 Mathis, Miles. The Compton Effect, Duality, and the Klein-Nishina formula. 2010.
8 Mathis, Miles. Three Problems Solved Mechanically. 2008.