return to homepage
return to updates
a clarification
by Miles Mathis

An astute and friendly reader contacted me recently, concerning my derivation of the angle of starlight curvature caused by the Sun. In a paper from 2005, I used expansion to solve this problem, achieving the same number as Einstein, about 1.7 arc seconds. I provided a diagram there, which I also used in my paper called “How to solve GR Problems without the Tensor Calculus, in 1/100th the time.” However, according to this reader, I failed to consider that the length of the AU would have to be increasing at the same time and same rate as the Sun and Earth radii were increasing: otherwise the Sun and Earth would be getting nearer.
The reader is absolutely correct in this. However, I will show that this fact changes nothing in my solution, since the operation that we use to calculate bending ignores this factor. The angle of bending is not the angle between “where we were at AU1” and “where we are at AU2.” The angle of bending is defined as the difference between “where we see the light on a photoplate” and “where we expected to see the light (from a starchart).” This is a bit tricky, so I will take you through it, starting with his diagram.
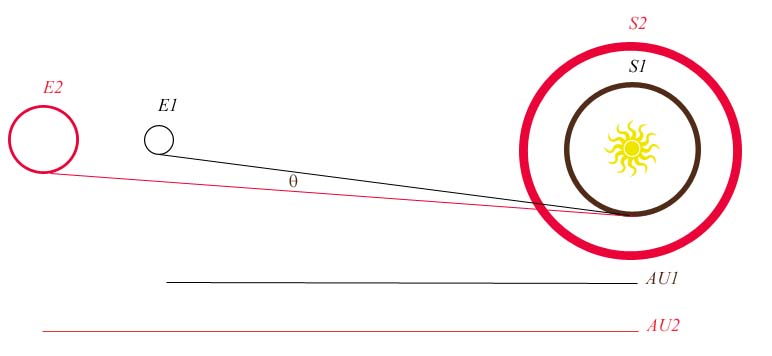
He lets everything expand at the same rate, so that, despite all the expansion, everything looks the same before and after. We have the appearance of stability, which matches the current data. But we have a variant explanation of gravity, with motion as the cause of all effects, not force.
So far so good, but let us see what his diagram does to the math. If we let the time between frame 1 and frame 2 be 500 seconds, then the length of the red line AU2 is no longer our current number, which means that the light should take longer than 500 seconds to travel to E2.
The first thing we have to do is find AU2. In 500 seconds, at 9.8 m/s2, the Earth radius increases 1,225,000m. That is an increase of 1.192x. To maintain the appearance of size stability, the AU must increase by that amount also. So, AU2 = 1.788 x 1011 m. It would take light an extra 96 seconds to travel that far (if light traveled in that way—but it doesn’t).
To find the angle, we have to do a bit more math than we did before.

tanφ
2 = opp/adj = AU2/(rS - rE2)
φ 2 = 89.77957 degrees
tanφ1 = AU1/(rS - rE1)
φ1 = 89.73678
θ = φ2 - φ1θ = 154 arc seconds
That is almost one hundred times bigger than Einstein’s angle, or my re-derivation of it. Why? Because that angle is not the angle we actually see when we see a star whose light appears to bent by the Sun.
In the diagram, says my reader, we think we are at E1 but we are really at E2, and this causes the calculation of bending. We think the angle belongs to the light, but it actually belongs to us. Well, kind of. The problem is, E1 and E2 exist at different times. As I showed with the math, E2 is 96 seconds after E1. But we have never had any idea that E1 exists, historically, so we could not take E1 as our “where we expected to see the light,” or “where we think we are.” We have no idea of a time or distance differential. In other words, we do not think we are at the position or time of E1, we only think we are at the radius of E1.
Therefore, to draw a diagram that correctly expresses “where we are” compared to “where we think we are,” we have to superimpose E1 and E2, as I did it originally. We can’t express the time differential, because there is no time differential between “now” and “now.” “Where we think we are” and “where we are” are both in the present: just look at the verbs. “Are” and “are.” Or, to use the other comparison, “where we see the light” and “where we expect to see the light” are also both now. The angle is bent compared to our expectation of it. It is bent compared to where we predicted the light would be, based on non-GR equations or non-expansion equations. Without expansion, no one would ever predict we would have a time or distance separation, so the position of E1 can’t enter the equations. Only the radius of E1 can enter the equations.
Therefore, my reader has not found Einstein’s angle. He has not found the angle we measure when we measure an angle on a photoplate and compare it to a starchart. I found that angle by my simple method of superimposing the two Earths, and now I hope you are better able to see why.
I have one other problem with my reader’s analysis here, and that is that he says that “space must also be expanding.” I cannot agree with that. I agree that the AU will be getting larger during the 500 seconds that light is traveling from the Sun to the Earth, but this is not due to any expansion of space. It is caused by a mutual repulsion between the Sun and Earth, due to the foundational E/M field. As I have shown in a series of other papers—where I rework the foundations of E/M—all objects must be repulsing each other, from the quantum level to the macro-level. This repulsion explains charge at the quantum level, and explains orbital stability at the macro-level.
Nor will the light take longer to travel AU2 than AU1. Remember Einstein's Postulate 2: nothing has a velocity relative to light. If we let light take longer to travel AU2 than AU1, then the Earth has a velocity relative to light during that interval. The way we think of this using expansion is that light is material, and therefore takes part in the expansion just like all the other objects. We cannot let everything else expand absolutely but keep keep light and c stable. The speed of light c is only stable relative to everything else. Our measurement of c cannot change, just as our measurement of 1 AU cannot change: and this is simply because we cannot measure our own expansion. All velocity is relative, and so is all distance. Relative to us, neither the AU nor c change. Therefore, we can say they are stable. We will always measure c as c. But if we look at light in these expansion diagrams, we must let it expand, too. These are diagrams that show absolute changes over time, and in them the photon must expand as a physical particle, and the speed of light must also increase. In an absolute sense, light is accelerating just like everything else. This is how we explained inertia, remember. Even things we think are absolutely still are accelerating in all directions. You are accelerating in all directions, and so are the table and the chair and the dog. If even still things are accelerating, then of course light must be accelerating, too.
If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.