return to homepage
return to updates
by Miles Mathis
milesmathis.com
email:mm@milesmathis.com
First posted December 27, 2008
Abstract: I show that mesons may be explained by stacked spins, without quarks or chromodynamics. I do a full analysis of the muon, the pion, the kaon, the tau, the D meson, the eta meson, the charmed eta meson, the bottom eta meson, the Z particle, the muon neutrino, and the tau neutrino. Also additional theory for neutrino oscillation.
I have promised an analysis of mesons and I now make good on that promise. To make the best speed through this paper, a reader should have already read my papers on superposition and on the electron/proton unification. He or she would also do well to read my paper on QCD.
In my superposition paper I first showed that any spherical particle could be shown to have four stacked spins, those being an axial spin plus the x, y, and z spins. Each spin is orthogonal to neighboring spins and outside the gyroscopic influence of other spins. Applying this simple analysis to the photon, I was able to explain the various mysteries of light propagation and interaction, including the the so-called paradoxes of detectors in sequence and so on. I dissolved the wave-particle duality, showing that it was explainable and visualizable in mechanical terms. This also dissolved the Copenhagen interpretation, which is now a relic.
In my paper critiquing QCD I showed that the neutron and the proton were the same particle, with different stacked spins. I showed that a neutron can be made into a proton simply by reversing its z-spin. This explains beta decay by a straightforward mechanical process.
In my paper unifying the electron and proton, I showed that a proton stripped of the three outer spins would have precisely the energy and mass of an electron at rest. This explained the number 1821 in a mechanical way, showing all the spin math.
Here I will show that mesons are protons or neutrons stripped of the z-spin, or electrons with superadded middle spins. I will show why mesons come in a variety of energies and how these energies are composed, using only stacked spins. I will also show why mesons are not stable.
In my paper unifying the electron and proton, I found a basic meson state at 1025/9 = 113.9 times the energy of the electron at rest. This is an electron with 3 spins, or a proton missing the z-spin. This gives us 113.9 x .511 MeV = 58.2 MeV. That is not the energy of any known meson, so my first task is explaining how to arrive at a known meson from this number.
The Muon
Let us start with the muon, with a mass of 105.7 MeV. The muon is no longer considered a meson, but it is actually the primary and fundamental meson. It is the most stable meson, and it is the most stable because it is the most fundamental. It is simply the electron with three spins. It lacks only the z-spin. If it had a z-spin, it would be a baryon. If you go to my paper on electron/proton unification, you will see that I have mathematically and mechically derived the values of the four fundamental spin levels, and the muon inhabits the third level, with no other complication but the one I will now explain.
The first thing to notice is that 105.7 is almost twice 58.2, so we will proceed by postulating that the muon is double the third energy level (for some reason yet to be discovered). Even if that is so, I still have too much energy in my derived and predicted number of 116.4, and that is because that number includes the emission field. In my paper on the electron/proton unification, I started at unity by assigning the non-spinning electron the number 1. I assigned no number to the emission field, so all my numbers in that paper included it. In other words, my foundational theory postulated the particle and its emission as a single number, but experiment measures only the particle and its spins. In experiment, the emission escapes into the field (except with the neutron and other neutral mesons that trap the field). The muon has a charge and is not neutral, therefore its emission is not measured to be part of its mass or energy in experiment. This is the difference between my initial number and the number of experiment.
Let us develop a number for that emission field. In my paper on QCD, I developed an energy for the summed emission field of the baryon. It is simply the mass difference between the proton and neutron. The neutron traps this energy and the proton emits it. This energy is in the amount of 2.31 x 10-30 kg. But this energy is a field, so it must be denser if emitted from a smaller radius. The y-level has half the radius of the z-level, so by the inverse square law the field will be 4 times as dense. Relative to the particle itself, it will not have gained mass or energy, but relative to a measurer, it will. An equivalent energy in a smaller space must appear to gain mass. For the same reason, the loss of the z-spin will double the mass of the field once more, since the z-spin was orthogonal to the y-spin. As we saw in the electron/proton unification paper, only half the z-spin multiplies with the y-spin. Not only the particle but also the emission must move against the linear motion half the time. We divided by 2 when we added the z-spin, so we must multiply by 2 when it is stripped away. This gives us a total increase of 8 times. [A shorter way to arrive at this 8 is to notice that the z level has 8 times the energy of the muon level (16385/2049). But here we are looking at the emission field, not the spins of the particle. The two fields act in a reverse way. The total energy of the particle increases as we go to outer spins. The energy of the emission field increases as we move in. The emission field becomes denser as we get nearer the surface of the particle, while the spins get more energetic as they gain more radius and torque. But both fields follow the same radius and change in the same way, in reverse. So if the spin field increases eight times, the emission field decreases eight times]. If we multiply 8 x 2.31 x 10-30, we get 1.848 x10-29, which is 20.29 x the electron mass, which is 10.37 MeV. If we subtract that amount of emission from my theoretical number, we get 116.3 - 10.37 = 105.9 That is near enough to the muon mass for my rough method here.
But why is there no neutral muon? By my mechanical theory of stacked spins, a neutral particle is a particle whose spins trap the emission. The neutron does this by creating a total spin path that sends the emission back to the particle (see illustration below). The emission cannot escape and becomes part of the mass of the particle. This is why the neutron weighs more than the proton. But the muon cannot create a path that comes back to the particle. We require four vectors for that, and the muon has only three. This is also why the muon has no stable state. The y-spin is the outer spin of the muon, and this spin must always be othogonal to the line of motion. The y-spin cannot match the linear motion, nor can it be opposite to it. So we cannot have a forward moving charge, creating stability, nor a reversed charge, creating neutrality. This missing neutral muon is convincing evidence in favor of my stacked spins. You can see that my theory has a simple mechanical explanation for it. Ask the standard model why there is no neutral muon.
Finally, why is the muon twice the third energy level, instead of inhabiting it exactly? I don't know at this time. It may be that the muon is actually two fundamental particles, this fundamental particle being the true meson. The muon may be a meson/anti-meson pair. Or, I may still have my 2's in disarray. My math contained a error of two (which is still in my book) and it may still contain one.
The Pion
Now let us look at the pion, another common meson. The energy of the pion is about 139.6 MeV. In my previous paper, I showed that the electron with axial spin only was the electron at rest (or low speed). To show a de Broglie wave motion, the electron had to move at a certain speed and experience collisions in the field. At this speed it would develop an x-spin and gain the energy of that spin. With both spins, the electron could express the wave in a simple mechanical way. I showed the energy of the electron with x-spin was 7.222 times the energy of the electron rest mass. I said that in this way the electron at speed was a sort of stable meson (since both x and y-spins could be called "mesonic" states). In the same way, I showed that the electron with y-spin would have 1025 its rest energy. The electron with axial spin was the rest energy. If we divide 2049 by 7.222 we obtain 283.7, which (multipying by .511MeV, the energy of the electron at rest) is 145 MeV. So, as a matter of stacked spins, the pion is the muon times 9 divided by 7.222. Mechanically, we may imagine that the pion is created by a collision orthogonal to the direction of linear motion, stripping the baryon of z and x spins simultaneously, but momentarily boosting the y-spin. This boost is achieved by a linkage between the y-spin and the axial spin, as we see from the math. This is not difficult to postulate or visualize, since without the intervening x-spin, the a and y vectors would naturally link. Nor is it difficult to imagine a collision that would target x and z levels, since they are also linked as vectors.
This would also explain why a pion quickly becomes a muon, and why the muon is more stable. The muon has all inner spins, at natural levels. It only lacks the z-spin. It has a normal level of charge protection, although this charge is moving orthogonally to the linear motion, and therefore cannot express its full character. The charge field also lacks a z-spin, stripping it of its largest defense. Therefore the muon is not stable. But the pion is even less stable, since it is missing the x-spin as well as the z-spin. It is momentarily more massive, since it has gained energy from the collision. But this mass cannot be maintained, since the fields that could absorb it are gone. The pion tries to rebuild as a muon, funneling energy down from y to x, but it cannot do so. As the pion collapses, it pauses momentarily at the muon energy while the rebuilding is attempted and failed, then, like the muon, it dissolves. It, like the muon, must dissolve either into an electron with axial spin, which we can detect; or into an electron with no spin, which we cannot detect. We will not detect the failed particle until it benefits from collision and re-establishes itself as a spin particle (or forms a multiple and becomes a neutrino—see below).
Now, we only need explain the difference between 145 and 139.6. Fortunately, this gap is the same relative size as my gap with the muon. We had a 10.37 MeV gap with the muon, and we have a 5.4 MeV gap with the pion. That is near enough to 2 for this paper. The pion is larger, so by my math and theory, we would expect a smaller gap. The gap is caused by the emission field, so how would we expect the emission of the pion to differ from that of the muon? The pion is missing the x-spin on the emission. This means that it is missing one of the 2's in the math. Even though the y and a-spins are augmented by the collision, the y-spin has no x-spin to double beneath it (see the full spin math in my previous paper for clarification here).
The biggest problem with the pion is found in the neutral pion. Experiment gives us an energy of about 135 for the neutral pion, and that cannot fit my theory at all, at first glance. I have proposed that neutral particles block emission, so that the mass of the emission must increase the mass of the particle. A neutral pion should act like a neutron, swallowing its own emission. This would make it weigh more. The neutron weighs more than the proton, not less. So the neutral pion should weigh more than the charged pions. A neutral pion should weigh 145, not 135. But this also has a simple mechanical explanation. Blockage of emission can only take place if the emission travels through the four spins and obtains a final trajectory that takes it back toward the particle. This is what happens with the neutron, as I showed in my analysis of beta "decay". But the pion cannot create this path. It lacks x and z spins. It has only a and y spins. Even though the a and y spins are augmented, they still cannot create the path in the right way. We have mechanical vectors at all points, with nothing esoteric or hidden, so if we want to explain a different energy, we must do so directly, with a clear visualization. The answer lies in the easily demonstrable fact that a particle with only a and y spins will funnel the emission back to the particle, but the emission will miss the particle (see illustration below). Therefore, the emission is in vector opposition to the linear motion of the particle, but it is not "re-absorbed" by the particle. If the particle is moving in the +a direction, the emission will be summing in the -a direction; but since it is missing the particle, its energy cannot be added to the total. Its energy must be subtracted from the total. This is why we subtract the 5 MeV instead of adding it. Instead of 140 + 5, we obtain 140 - 5, which gives us about 135.
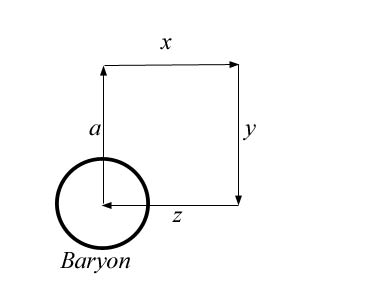
with only a and y vectors, the pion's emission misses the particle
(the particle is also moving +a)
[In imagining the loss of x and z vectors in the illustration above, you must not allow the a and y vectors to line up, so that y points right back at the particle. This is because this two-dimensional illustration is missing the third dimension. In a three-dimensional schematic, the a and y vectors would not in fact line up. The illustration here is a useful visualization in some ways, but it is not complete or completely accurate.]
The Muon Neutrino
I proposed above that muons and pions must dissolve either into electrons with axial spin or without. We know what an electron with axial spin looks like, since I have proposed that this is the normal electron at rest. But what is an electron with no spin? In my paper on electron/proton unification, I showed that the non-spinning electron would have a mass of 1/9 that of the spinning electron. But do we ever find such a particle? We wouldn't expect to find it by normal methods, since the normal methods use E/M fields, and the non-spinning electron would have neither charge nor magnetism. But watch this: If we divide the current energy of the electron .51MeV by 9, we obtain .05678., which is 56.78 keV. Still not ringing a bell? Well, the energy of the muon neutrino is 170keV. If we divide 170 by 56.78, we get exactly 3. There is no muon neutrino. The muon neutrino is three non-spinning electrons huddling together for protection, each one trying to block the charge wind in one of the 3 dimensions. These electrons have no charge or emission, so they do not repel eachother. They cannot repel any other particles either, so they get pushed into groups like this, where their only repulsion is from the strength of their shells or surfaces.
All neutrinos may be explained by other means. Here I have shown that the muon neutrino is three non-spinning electrons in a group. In my QCD paper, I showed that the electron neutrino was a variation in the charge field caused by a z-spin reversal. The tau neutrino will be lost below in the same way, to a simple mechanical explanation.
Neutrino "Oscillation"
I showed at the end of my QCD paper that neutrino oscillation is actually a change in the B-photon or emission field, not the reincarnation of one particle to another. But here I give the muon neutrino to an electron triplet. Which is it? Both. In neutrino oscillation we go from one unseen flux to another. We call the first flux an electron neutrino and the second a muon neutrino. But in reality, the first flux is a flux of the B-photon field, with no neutrino present. The second is a flux of the electron field, with electrons colliding with free neutrons and losing their axial spins. Again, there is no neutrino present, but there is a new particle present after the second event: an electron triplet. In each stripping, a neutron is stripped of its z-spin and multiple electrons are stripped of their axial spins. It would appear that it takes three electrons to strip each neutron. The axial spin has a much lower energy than the z-spin, and only by adding their linear energy to the equation can the moving electrons successfully attack a neutron. I will have more to say about this in other papers.
The Tau Neutrino
The tau neutrino has been called a neutrino simply because the standard model had no other way of categorizing such a small meson. The tau neutrino has an energy of 15.5 MeV. That is about 30 times the energy of the resting electron. If we bring an electron up to speed, it will gain an x-spin and thereby a wave. I have shown that this x-spin will give the electron 7.222 times the energy of the resting electron, which is 3.69 MeV. You can see from both these comparisons that the tau is about 4 times the x-spinning electron. So it turns out that the tau is another multiple: it is four x-spinning electrons. But why would x-spinning electrons join in groups of four?
As it turns out, the x-spinning electron has a number of states, and only one of these states is stable. That is the electron we measure when we measure an electron with a wave. In this state, the emission is summing in the same direction as the linear motion. To say it another way, the linear vector and the x-vector match. This gives the moving electron a protective charge field in front with its full numerical value, and allows the electron to repel other electrons.
But this is only one of several possible states. In all analyses up to now, I have assumed that the electron had only four total vectors, and I have implicitly let the linear vector match the axial vector. But now I must include the further complication that the axial and linear vectors may be orthogonal. If this is the case, we will have to analyze not four but five orthogonal vectors in every baryon. For instance, let the electron spin about a y-axis. The emission will be thrown out mainly in the xz plane. If we let the electron now move in x or z, then the linear motion and the emission will match, in a vector sense. But if we let the electron move linearly in y, it will be moving orthogonally to its own emission, you see. It would not be stable, because it would have no protection from the emission. This unstable x-electron is the component of the tau neutrino.
To say it another way, the particle is moving forward and emitting sideways. The x-spin is unstable, and would tend to decay back to the axial level or lower. This is how we get the tau neutrino. The tau neutrino is four x-spinning electrons, all of the unstable variety, and all different from the others. By setting themselves up this way, the unstable x-spinning electrons are able to to become stable. The tau neutrino is emitting up, down, and to both sides, and has a charge weakness only directly ahead of it, where there is a minima. For this reason, it is stable, but not as stable as an electron. I have called all these constituents of the tau neutrino electrons, but I think it is clear that two of them are positrons. To be precise, I should say that the tau neutrino is made up of two electron/positron pairs, orthogonal to one another.
I should also come up with a better name for the tau neutrino, since I have now shown that it is not a neutrino. There are no neutrinos. I will call it the quirino for now. In honor of Majorana, who came so close to solving the problem of gravity. It is close to quarto, but I don't want quarto since it is already overused.
The Kaon
In experiment we find that a kaon in decay can yield either pions or muons. This was the hint I needed to discover its construction. The most stable kaon is very long-lasting, living for some 10-8 seconds, and weighing about 493.7 MeV. Two charged pions and two muons give us almost 492 MeV. The long life is given it by the muons. They add stability to the kaon, making it much more stable than other mesons composed only of pions (such as the eta meson, below, which lives only 10-19 s). Pions and muons both emit sideways, which allows them to huddle in groups of four, either with one another or with with themselves. We have four charge orientations at the y-level, and one particle in the quadruplet represents each orientation. The kaon therefore emits to all four sides, missing only the forward emission (relative to its linear motion). The lack of forward emission makes the kaon a meson, and keeps it from having stability. But the partial muon construction protects it in collision, since the muon has all inner spins.
The Eta Meson
The eta meson is clearly just 4 pions. It is either 3 neutral pions and a charged pion, or 2 neutral pions and 2 charged pions. This would give us either about 545 MeV or 550 MeV, depending on the combination. The eta meson is 547.8 MeV in experiment. Currently the eta is said to decay into three pions, but the trackers are missing one of the pions, due to the orthogonal vectors. It is almost impossible to track orthogonal vectors simultaneously in such a complex decay.
Charmed Eta Meson
The charmed eta meson is another meson that matches my equation outputs almost precisely. You will have noticed that my fundamental meson states are all multiples of 2. The charmed eta resides near this level: [1 + (8 x16 x 32 x 64 x 128)/29] = 65,536. We divide by 9 to achieve a multiple of the electron, and then multiply by .511, which gives us 3721 MeV. As with the pion, we only need one more factor, and that is 7.2222/9 (see above). This gives us 2986 MeV. The charmed eta meson in experiment is 2980. From this we may conclude that the charmed eta is related to the pion, each of them losing inner spins in collision.
Bottom Eta Meson
The bottom eta is another meson created like the pion and charmed eta. We have a fundamental meson level at [1 + (8 x16 x 32 x 64 x 128)/28] = 131,072. If we divide by 9, we obtain 14,560, and if we multiply by .511, we get 7,442 MeV. If we multiply by 9/7.222, we obtain 9273 MeV. The experimental value of the bottom eta is 9300 ± 40. Like the charmed eta and the pion, the bottom eta is missing inner spins after a collision from the side.
The Tau
The tau has been a very difficult particle to isolate in experiment, and that is because it is a complex combination of mesonic states. It decays into at least one muon, and this fact helped me a bit to discover its make-up. As I showed above, pions commonly decay into muons, as they shed unstable outer spins. So we should look for the pion in the tau. The tau was also a difficult particle for me to explain, since it is the first example I uncovered of a baryon huddling with a meson. In this case, we have two unstable baryons huddling with two pions.
Let me first explain the unstable baryon. In my QCD paper I offered 16 baryon states, assigning eight to neutrons/anti-neutrons and eight to protons/anti-protons. This was a complete list of stable baryons, but it is not a complete list of baryons. We have 16 more unstable baryons. The mechanical reason for this goes back to my analysis of the tau neutrino, where I show that the emission field of any emitting particle may be emitted sideways. I showed that if we include the linear motion as a fifth vector, this vector may be orthogonal to the outermost spin. Since the spin does not emit in the same direction as the linear motion, it does not provide full charge protection. So the baryon may be emitting to any of four sides, all of them orthogonal to the linear motion. If we combine these z-spins with inner spins, we obtain 16 more states. All of these 16 are unstable. This gives us a total of 32 baryon states, and that number can be arrived at easily by summing the five possible vectors. With five vectors, we must have 25 possible states.
The tau makes use of two of these unstable baryon states. You may imagine that these two opposite baryons meet back to back, trapping two pions in the other corners. In other words, if the baryons are emitting north and south, the pions emit east and west. This gives the tau a split second of stability, until the baryons begin to turn and face eachother. The intervening pions prevent this turn for an instant, but they are overwhelmed by the energy of the baryons, and the structure quickly collapses. The pions become muons, and the baryons strip eachother of spin completely, decaying back to spinless electrons, and becoming invisible to our detectors.
But there is one other complication. The baryons are doubly unstable, due to a recent collision. Just as pions are damaged particles, lacking inner x-spins, the baryons in the tau are damaged baryons. They have been hit from the side, losing this same x-spin. It is as if the particle has been crushed in a vise, by a field orthogonal to its main motion. This orthogonal field targets inner spins. So we multiply by that same term we have seen before with the pion: 7.222/9. This term express a loss of the x-spin, and a linkage of the a and y spins. So take the baseline energy for the baryon [1 + (8 x16 x 32 x 64)/24] = 16,384, divide by 9 to find a multiple of the electron and multiply by .511. This gives us 930 MeV. Then we multiply by 7.222/9, to express the collision or vise. This gives us 746.6 MeV. Doubling that to express two particles gives us 1493. Two charged pions gives us 279.2. Adding them all together, we have 1772. The energy in experiment of the tau is 1777.
To sum up, we have two baryons and two pions, both damaged from the same sideways field or collision, and all missing the x-spin. They combine in a NSEW square, and then quickly decay.
The Z particle
Using my meson equation, we can find a value for the Z. The Z is a baryon with several unstable spins forced on top of the stable z-spin. It is always found in high-energy accelerators, so it must be a product of collision. I have rewritten this section based on later calculations I did in my Higgs papers of 2012. It is my current opinion that the Z is three particles in collision, all of them multiples of the proton. Here is the primary equation: [1 + (8 x16 x 32 x 64 x 128 x 256)/214] = 524,289. That is very nearly 219. We then divide by 9 to achieve a multiple of the electron, and then multiply by .511, which gives us 29.8 GeV. If we double that again, we get 59.5GeV. If we divide the first number by 16, we get 1.86GeV. If we add them all together, we get 91.16GeV, which is the energy in accelerator of the Z. This means that the Z is a level 20 proton meeting a level 19 proton and a level 15 proton. This analysis overwrites my previous analysis.
The D meson
"Meson" is not a logical term for a particle above the baryon mass, so I will call these particles "uberons." The D meson is another fundamental level predicted by my math. We use this equation: [1 + (8 x 16 x 32 x 64 x 128)/210] = 32,768. Dividing by 9 and multiplying by .511 gives us 1860.5 MeV. The D meson in experiment is about 1865. The math shows us that the D meson is a baryon with an added spin above the z-spin. We can add either a second x or a second y level above the z level, with one giving us 1860.5 MeV and the other giving us 7442 MeV (see the bottom eta). At 7442 MeV, the numerator is the same, but the denominator will be 28. The number of 2's depends on whether the outer spin is "doubling" the inner x or inner y level. One will be orthogonal twice and the other will be orthogonal three times, so the denominator must represent this inner doubling.
Other mesons and uberons
Several other mesons and uberons can be explained as multiples of the ones above. Using the tools I have created in this paper, we can find the structure of any meson or uberon. For instance, the vector B meson is just three taus. We can see this immediately from the energies. The rho meson is one baryon without x-spin and two tau neutrinos. The strange D meson is four kaons.
The charmed B meson is the first uberon state (7442 MeV) without inner x-spin, plus two pions. That gives us 5973 + 140 + 140 = 6253. The charmed B in experiment is 6275. Once again we have the baryon and pions missing the same x-spin, from the same sideways field.
The so-called vector mesons have much shorter lives than most of the others, and this is because they are often initial states of pseudoscalar mesons. The vector meson decays into the pseudoscalar meson on the way down. This does not mean that the vector mesons are taking part in quark bonding or anything else. They are not bosons. They are simply more complex multiples of the basic states shown above, which makes them more unstable and often larger.
Conclusion
Perhaps after all this you can see that the ad hoc quantum numbers of QCD are no longer needed. CP parity, for instance, is completely explained by stacked spins. We don't have "vector" mesons or "pseudoscalar" mesons, we simply have mesons and uberons. Inner spins give us a simple mechanical and visualizable explanation of parity, as well as all the other quantum numbers like spin, isospin, and flavor. My theory will do a housecleaning not only on the particles, but on the names. We can now jettison most of the useless and unmechanical vocabulary of atomic physics. In upcoming papers I will suggest simpified terminology to go with our new simplified physics.
If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.